









By QB365 on 31 Dec, 2022
QB365 provides a detailed and simple solution for every Possible Questions in Class 11 Chemistry Subject - Important 5 Mark English Medium. It will help Students to get more practice questions, Students can Practice these question papers in addition to score best marks.
11th Standard
Chemistry
Answer All The Questions
Mass of one atom of an element is 6.645 x 10-23g. How many moles of element are there in 0.320 kg.
Balance the following equations by ion electron method.
i) \({ KMn }O_{ 4 }+{ SnCl }_{ 2 }+HCI\longrightarrow MnCI_{ 2 }+{ SnCI }_{ 4 }+{ H }_{ 2 }O+KCI\)
ii)
iii)
iv)
Balance the following equation using oxidation number method
As2 S3 + HNO3 + H2O \(\rightarrow\) H3 AsO4 + H2 SO4 + NO
State and explain pauli exclusion principle.
i) Describe the Aufbau principle.
ii) What is effective nuclear charge ?
Explain the pauling method for the determination of ionic radius.
How do you convert para hydrogen into ortho hydrogen?
Explain the important common features of Group 2 elements.
Distinguish between diffusion and effusion.
Define the following terms
(a) isothermal process (b) adiabatic process
(c) isobaric process (d) isochoric process
List the characteristics of Gibbs free energy
Calculate the enthalpy change for the reaction
Fe2O3 + 3CO ⟶ 2Fe + 3CO2 from the following data.
2Fe +\(\frac{3}{2}\)O2 ⟶ Fe2O3; ΔH = -741 kJ
C +\(\frac{1}{2}\)O2 ⟶ CO; ΔH = -137 kJ
C + O2 ⟶ CO2; ΔH = - 394.5 kJ
When I-pentyne (A) is treated with 4N alcoholic KOH at 175°C, it is converted slowly into an equilibrium mixture of 1.3% I-pentyne(A) , 95.2% 2-pentyne(B) and 3.5% of 1,2 pentadiene (C) the equilibrium was maintained at 175°C, calculate ΔG0 for the following equilibria.
B \(\rightleftharpoons \)AGIO?
B \(\rightleftharpoons \)CG20?
The equilibrium constant at 298 K for a reaction is 100.
A + B \(\rightleftharpoons \) C + D
If the initial concentration of all the four species is 1 M, the equilibrium concentration of D (in mol lit-1) will be
State and explain Henry’s law.
Explain resonance with reference to carbonate ion ?
Explain VSEPR theory. Applying this theory to predict the shapes of IF7, and SF6.
Describe the reactions involved in the detection of nitrogen in an organic compound by Lassaigne method.
Identify the compound A, B, C and D in the following series of reactions

Differentiate the following
(i) BOD and COD
(ii) Viable and non-viable particulate pollutants
Arrange the elements silver, Zinc and copper in the order of their decreasing electron releasing tendency and justify your arrangement with an appropriate experiment.
Balance the following equations by oxidation number method.
P + HNO3 ⟶ HPO3 + NO + H2O
Give the chemical properties of heavy water and water.
Explain the preparation and uses of the following compounds of calcium.
Explain P-V relationship experiments of Robert Boyle.
If a scuba diver takes a breath at the surface filling his lungs with 5.82 dm3 of air what volume will the air in his lungs occupy when he drives to a depth where the pressure is 1.92 atm. (assume temperature is constant and the pressure at the surface is exactly)
At sea level a balloon has volume of 785 x10-3dm3 What will be its volume, if it taken to a place where the pressure is 0052 atm. Less than the atmospheric pressure of 1 atm.
The critical temperature of hydrogen gas is 33.2 °C and its critical pressure is 12.4 atm. Find out the values of a and b.
Explain Andrew's isotherm of carbon dioxide.
Calculate the entropy change in the engine that receives 957.5 kJ of heat reversibly at 110°C temperature.
Answers
mass of one atom = 6.645 x 10-23 g
\(\therefore\) mass of 1 mole of an atom = 6.645 x 10-23 g x 6.022 x 1023 = 40 g
\(\therefore\) number of moles of element in 0.320 kg = \(\frac { 1\quad mole }{ 40g } \times 0.320kg\)
= \(\frac { 1mol\times 320g }{ 40g } \)
= 8 mol
Half reaction are:
\(\overset { +7 }{ M } { nO }_{ 4 }^{ - }\longrightarrow { Mn }^{ 2+ }\)
and \({ Sn }^{ 2+ }\longrightarrow { Sn }^{ 4+ }\)
(1) \(\Rightarrow \) \({ MnO }_{ 4 }^{ - }+{ 8H }^{ - }+5e^{ - }\longrightarrow { Mn }^{ 2+ }+{ 4H }_{ 2 }O\)
(2) \(\Rightarrow \) \({ Sn }^{ 2+ }\longrightarrow { Sn }^{ 4+ }+{ 2e }^{ - }\)
.png)
ii)
iii)
iv)
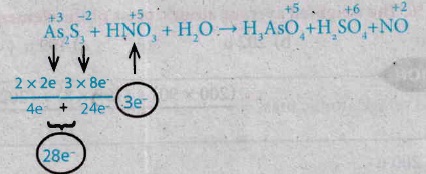
Equate the total no. of electrons in the reactant side by cross multiplying.
\(\Rightarrow 3 \mathrm{As}_{2} \mathrm{~S}_{3}+28 \mathrm{HNO}_{3}+\mathrm{H}_{2} \mathrm{O} \rightarrow \mathrm{H}_{3} \mathrm{AsO}_{4}+\mathrm{H}_{2} \mathrm{SO}_{4}+\mathrm{NO}\)
Based on reactant side, balance the products
\(\Rightarrow 3 \mathrm{As}_{2} \mathrm{~S}_{3}+28 \mathrm{HNO}_{3}+\mathrm{H}_{2} \mathrm{O} \rightarrow 6 \mathrm{H}_{3} \mathrm{AsO}_{4}+9 \mathrm{HSO}_{4}+28 \mathrm{NO}\)
Product side : 36 hydrogen atoms & gg Orygen atoms
Reactant side : 28 hydrogen atoms & 74 Orygen atoms
Difference is 8 hydrogen atoms & 4 oxygen atoms
∴ Add 4 H2O molecule on the reactant side.
Balanced equation is,
\(3 \mathrm{As}_{2} \mathrm{~S}_{3}+28 \mathrm{HNO}_{3}+4 \mathrm{H}_{2} \mathrm{O} \rightarrow 6 \cdot \mathrm{H}_{3} \mathrm{AsO}_{4}+9 \mathrm{H}_{2} \mathrm{SO}_{4}+28 \mathrm{NO}\)
Statement : "No two electrons in an atom can have the same set of values of all four quantum numbers"
Explanation : It means that, each electron must have unique values for the four quantum numbers (n, l, m and s).
For the lone electron present in hydrogen atom, the four quantum numbers are: n = 1; l = 0; m = 0 and s = +1/2. For the two electrons present in helium, one electron has the quantum numbers same as the electron of hydrogen atom, n = 1.
l = 0, m = 0 and s = +1/2. For other electron, the fourth quantum number is different i.e., n = 1, l = 0, m = 0 and s = -1/2.
As we know that the spin quantum number can have only two values +1/2 and - 1/2, only two electrons can be accommodated in a given orbital in accordance with pauli exclusion principle.
| Atom | e- | n | l | m | s |
| Helium | First | 1 | 0 | 0 | +1/2 |
| Second | 1 | 0 | 0 | +1/2 |
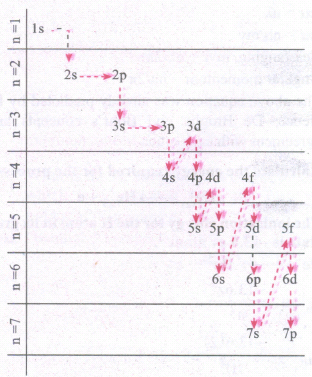
The word Aufbau in German means 'building up'. In the ground state of the atoms, the orbitais are filled in the order of their increasing energies. That is the electrons first occupy the lowest energy orbital available to them.
Once the lower energy orbitals are completely filled, then the electrons enter the next higher energy orbitals. The order of filling of various orbitals as per the Aufbau principle which is in accordance with (n + l) rule.
(i) Ionic radius of uni-univalent crystal can be calculated using Pauling's method from the inter ionic distance between the nuclei of the cation and anion.
(ii) Pauling assumed that ions present in a crystal lattice are perfect spheres, and they are in contact with each other therefore,
d=rC+ + rA- ...(1)
Where d is the distance between the centre of the nucleus of cation C+ and anion A-and rC+, rA- are the radius of the cation and anion respectively.
(iii) Pauling also assumed that the radius of the ion having noble gas electronic configuration is inversely proportional to. the effective nuclear charge.
\({ r }_{ C }^{ + }\alpha \frac { 1 }{ ({ Z }_{ eff }){ C }^{ + } } \) ....(2) and
\({ r }_{ A }^{ - }\alpha \frac { 1 }{ ({ Z }_{ eff }){ A }^{ - } } \)...(3)
Where Zeff is the effective nuclear charge and Zeff= Z - S
Dividing the equation 1 by 3
\(\frac { { r }_{ C }^{ + } }{ { r }_{ A }^{ - } } =\frac { ({ Z }_{ eff }){ A }^{ - } }{ ({ Z }_{ eff }){ C }^{ + } } \) ...(4)
On solving equation and (1) and (4) the values of rC+ and rA- can be obtained.
(i) By treatment with catalyst like Pt or Fe.
(ii) By passing an electric discharge
(iii) By heating to 800°C or more.
(iv) By mixing with paramagnetic molecules like O2,NO,NO2·
(v) By mixing with nascent hydrogen or atomic hydrogen.
1. This group contains Be, Mg, Ca, Sr, Ba & Ra.
2. Except Be all these elements are called as alkaline earth metals because their oxides and hydroxides are alkaline in nature.
3. Beryllium is the rare element and Radium is the rarest (10% rocks) ; Their Occurrence: Be- Beryl; Mg - carnalllite; Dolomite Ca - Fluorapatite; Sr - Celestite Ba - Barytes.
4. Radium is radioactive.
5. The general electronic configr.ration is :
[Noble gas] ns2 eg. Be - [He] 2s2
6. On moving down the group the radii increase. Their atomic radii are smaller than alkali metals.
7. They exhibit +2 oxidation state.
8. The ionisation enthalpies are less than p - block elements due to large size. Down the group the ionisation enthalpy decreases.
9. The IE1 of group 2 elements are greater than group 1 elements.
10. The IE2 values are higher than that of alkalimetals.
11. They are less etectro Positive elements than alkali metals.
12. The hydration enthalpy decreases with increase in ionic radii.
13. MgCl2 form MgCI2 .6H2O and CaCl2 form CaCl2.6H2O
14. The electronegativity value decreases down the group.
15. With concentrated HCI They impart flame colour. Ca - Brick Red ; Sr - Crimson red and Barium - AppIe Sreen.
16. All form metallic halides at elevated temperatures. M + X2 + MX2
17. All erements except Beryllium combine with hydrogen to form hydrides of formula MH2.
| S.NO | diffusion | effusion |
| 1 | It is the spreading of molecules of a substance throughout a space or second substance | It is the escape of the gas molecules through a very small hole (orifice) in a membrane into an evacuated area. |
| 2 | It is typically used to describe statistical properties of a gas an length scales that are much larger than mean free path. | The diameter of the hole should be smaller than mean free path of the molecules. |
| 3 | It is the spreading of gases. | It is the pouring out of gases. |
(a) Isothermal process: An isothermal process is defined as one in which the temperature of the system remains constant, during the change from its initial to final state. The system exchanges heat with its surroundings and the temperature of the system remains constant.
For an isothermal process dT = 0
(b) Adiabatic process: An adiabatic process is defined as one in which there is no exchange of heat (q) between the system and surrounding during the process. For an adiabatic process q = 0
(c) Isobaric process: An isobaric process is defined as one in which the pressure of the system remains constant during its change from the initial to final state. For an isobaric process dP = 0 .
(d) Isochoric process: An isochoric process IS defined as the one in which the volume of system remains constant during its change from initial to final state. For an isochoric process, dV= 0.
(i) Free energy is defined as G = H - TS. 'G' is a state function.
(ii) G- Extensive property; ΔG - intensive property. When mass remains constant between initial and final states of system.
(iii) 'G' has a single value for the thermodynamic state of the system.
(iv) G and ΔG values correspond to the system only.
| Process | Spontaneous | Equilibrium | Non-Spontaneous |
| ΔG | -Ve | Zero | +Ve |
(v) Gibbs free energy and the net work done by the system:
For any system at constant pressure and temperature
ΔG = ΔH - TΔS .....(1)
We know that,
ΔH = ΔU + PΔV
ΔG =ΔU + PΔV-TΔS
from first law of thermodynamics
ΔU = q +w
from second law of thermodynamics
Δ S=\(\frac{q}{T}\) Δ G=q+w+PΔ V-T\((\frac{q}{T}) \)
Δ G = w+PΔV
-ΔG = -w - PΔV ......(2)
But -PΔV represents the work done due to expansion against a constant external pressure.
ΔHf(Fe2O3)= -741 kJ mol-1
ΔHf(CO)= -137 kJ mol-1
ΔHf(CO2)= -394.5 kJ mol-1
Fe2O3 + 3CO ⟶ 2Fe + 3CO2 ΔHr=?
ΔHr=Σ(ΔHf)products - Σ(ΔHf)reactants
ΔHr=[2ΔHf=(Fe)+3ΔHf(CO2)]-[ΔHf(Fe2O3)+3ΔHf(CO)]
ΔHr=[0 + 3 (-394.5)] - [-741 +3 (-137)]
ΔHr=[-1183.5] - [-1152]
ΔHr=-1183.5 + 1152
ΔHr=-31.5 kl mol-1
T=1750 C=175+273=448K
Concentration of l-pentyne [A] = 1.3%
Concentration of2-pentyne [B] = 95.2%
Concentration of 1, 2-pentadiene [C] = 3.5%
At equilibrium
B\(\rightleftharpoons \)A
95.2%1.3%\(\Rightarrow \)
\({ K }_{ 1 }=\frac { 1.3 }{ 95.2 } =0.0136\)
B\(\rightleftharpoons \)C
95.2%3.5%\(\Rightarrow \)
\({ K }_{ 2}=\frac { 3.5 }{ 95.2 } =0.0367\)
\(\Rightarrow \)\(\Delta { G }_{ 1 }^{ 0 }\) =-2.303RT log K1
\(\Delta { G }_{ 1 }^{ 0 }\)=-2.303X8.314X448Xlog0.0136
\(\Delta { G }_{ 1 }^{ 0 }\) =+16010J
\(\Delta { G }_{ 1 }^{ 0 }\)=+16 kJ
\(\Rightarrow \)\(\Delta { G }_{ 2 }^{ 0 }\)=-2.303X8.314X448Xlog0.0367
\(\Delta { G }_{ 2 }^{ 0 }\) =+12312J
\(\Delta { G }_{ 2 }^{ 0 }\)=+12.312 kJ.
Given data:
[A] = [B] = [C]= [D] =1 M
Kc = 100
[D]eq = ?
Solution:
Let x be the no moles of reactants reacted
| A | B | C | D | |
|---|---|---|---|---|
| Initial concentration | 1 | 1 | 1 | 1 |
| At equilibrium (as per reaction stoichiometry) |
1-x | 1-x | 1-x | 1-x |
\(K_c={[C][D]\over [A][B]}\)
\(100={(1+x)(1+x)\over (1-x)(1-x)}\)
\(\sqrt{100}=\sqrt{{(1+x)(1+x)\over (1-x)(1-x)}}\)
\(10={1+x\over 1-x}\)
10(1 - x) = 1 + x
10 - 10x - 1 - x = 0
9 - 11x = 0
11x = 9
\(x={9\over 11}=0.818\)
[D]eq = 1+x = 1 + 0.818 = 1.818M.
Henry's law states that, "the partial pressure of the gas in vapour phase is directly proportional to the mole fraction(x) of the gaseous solute in the solution at low concentrations".
Henry's law can be expressed as,
\(\rho \)solute \(\alpha\) X solute in solution
Psolute = KHx solute in solution
Explanation: Here, Psolute represents the partial pressure of the gas in vapour state which is commonly called as vapour pressure. x solute in solution represents the mole fraction of solute in the solution. KH is a empirical consiint with the dimensions of pressure. The value of 'KH' depends on the nature of the gaseous solute and solvent. The above equation is a straight-line in the form of y = mx. The plot partial pressure of the gas against its mole fraction in a solution will give a straight line as shown in fig The slope of the. straight line gives the value of KH.

Limitation of Henry's law:
i) Henry's law is applicable at moderate temperature and pressure only.
ii) Only the less soluble gases obeys Henry's law.
iii) The gases reacting with the solvent do not obey Henlry s law For example, ammonia or HCI reacts \Mith water and hence does not obey this law.
\(\mathrm{NH}_3+\mathrm{H}_2 \mathrm{O} \leftrightarrows \mathrm{NH}_4^{+}+\mathrm{OH}^{-}\)
(iv) The gases obeying Henry's law should not associate or dissociate while dissolving in the solvent.
'When we write Lewis structures for a molecule, more than one valid Lewis structures are possible in certain cases. For exampte let us consider the Lewis structure of carbbnate ion [CO3]2- . The skeletal structure of carbonate ion (The oxygen atoms are denoted as OA, OB & Oc
2. Total number of valence electrons = [1 x 4(carbon)] + [3 x 6 (oxygen)] + [2 (charge)] = 24 electrons.
3. Distribution of these valence electrons gives us the following structure.
4. Complete the octet for carbon by moving a lone pair from one of the oxygens (OA) and write the charge of the ion (2-) on the upper right side.
5. In this case, we can draw two additional Lewis structures by moving the lone pairs from the other two oxygens (OB & Oc) thus creating three similar structures as shown below in which the relative position of the atoms are same. They only differ in the position of bonding and lone pair of electrons. Such stnictures are called resonance structures (canonical structures) and this phenomenon is called resonance.
6. It is evident from the experimental results that all carbon-oxygen bonds in carbonate ion are equivalent. The actual structure of the molecules is said to be the resonance hybrid, an average of these three resonance forms. It is important to note that carbonate ion does not change from one structure to another and vice versa. It is not possible to picturise the resonance hybrid by drawing a single Lewis structure. However, the following structure gives a qualitative idea about the correct structure
7. It is found that the energy of the resonance hybrid (structure 4) is lower than that of all possible canonical structures (Structure 1, 2 & 3). The difference in energy between structure 1 or 2 or 1 3, (most stable canonical structure) and structure 4 (resonance hybrid) is called resonance energy.
Important principles of VSEPR Theory are as follows:
1. The shape of the molecules depends on the number of valence shell electron pair around the central atom.
2. There are two types of electron pairs namely bond pairs and lone pairs. The bond pair of electrons are those shared between two atoms, while the lone pairs are the valence electron pairs that are not involved in bonding.
3. Each pair of valence electrons around the central atom repels each other and hence, they are located as far away as possible in three dimensional space to minimize the repulsion between them.
4. The repulsive interaction between the different types of electron pairs is in the following order.
lp - lp > lp - bp > bp - bp
lp - lone pair; bp - bond pair
The lone pair of electrons are localised only on the central atom and interacts with only one nucleus whereas the bond pairs are shared between two'atoms and they interact with two nuclei. Because of this the ione pairs occupy more space and have greater repulsive Power than the bond Pairs in a molecule.

.jpg)
A small piece of Na dried by pressing between the folds of a filter Paper is taken in a fusion tube and it is gently heated.
When it melts to a shining globule, put a pinch of the organic compound on it. Heat the tube till reaction ceases and becomes red hot. Plunge it in about 50 mL of distilled water taken in a china dish and break the bottom of the tube by striking against the dish. Boil the contents of the dish for about 10 mts and filter. This filtrate is known as lassaignes extract or sodium fusion extract and it used for detection of nitrogen, sulfur and halogens present in organic compounds.
If nitrogen is present it gets converted to sodium cyanide which reacts with freshly prepared furro,sulphate and feiric ion followed by conc. HCI and gives a Prussian blue color or green color precipitate. It confirms the presence of nitrogen. HCI is added to dissolve the lreenish precipitate of ferrous hydrbxide iroduced by the excess of NaOH on Feson which would otherwise markthe Prussian blue piecipitate. The following reaction takes part in the formation of Prussian blue.
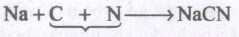
from organic compounds
\(FeSo_{ 4 }+2NaOH\longrightarrow Fe(OH)_{ 2 }+Na_{ 2 }{ SO }_{ 4 }\)
from organic compounds
\(6FeCN+Fe(OH)_{ 2 }\longrightarrow Na_{ 4 }[Fe(CN)]_{ 6 }+2NaOH\)
Sod.ferrocyanide
\(3Na_{ 4 }[Fe(CN)_{ 6 }]+FeC1_{ 3 }\longrightarrow Fe_{ 4 }[Fe(CN)]_{ 3 }+12NaCI\)
ferric ferrocyanidePrussian blue or greenppt
Incase if both N & S are present, a blood red color is obtained due to the following reactions.
\(\mathrm{Na}+\mathrm{C}+\mathrm{N}+\mathrm{S} \stackrel{\text { Heat }}{\longrightarrow} \mathrm{NaCNS}\)
sodium sulphocyanide
\(3 \mathrm{NaCNS}+\mathrm{FeCl}_3 \longrightarrow \mathrm{Fe}(\mathrm{CNS})_3+3 \mathrm{NaCl}\)
ferric sulphocyanide
(Blood red colour).
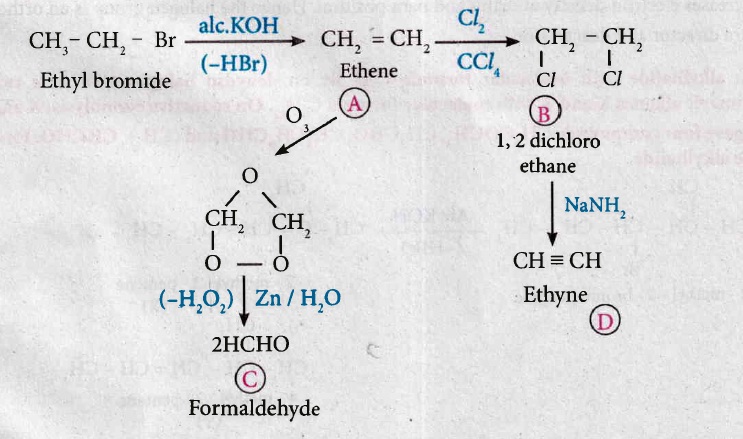
| compound | Structural formula | Name |
| A | CH2 = CH2 | Ethene |
| B | 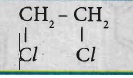 |
1,2 - dichloroethane |
| C | HCHO | Methanal |
| D | \(\mathrm{CH} \equiv \mathrm{CH}\) | Ethyne |
(i) BOD and COD
| No | BOD | COD |
| 1 | This is Bio chemical oxygen demand | This is chemical oxygen demand |
| 2 | The total amount of oxygen in milligrams consumed by microorganisms in decomposing the waste in one litre of water at 20o for a period of 5 days is called biochemical oxygen demand (BOD) |
Chemical oxygen demand (COD) is defined as the amount of oxygen required by the organic matter in a sample of water for its oxidation by strong oxidising agent like K2Cr2O7 in acid medium for a Period of 2 hrs. |
| 3 | It is expressed in ppm | It is expressed in mg/L |
| 4 | It is a measure of consumed oxygen | It isa measurement of requirement of dissolved oxygen. |
| 5 | In waste streams BOD levels are less than COD. | In waste streams COD levels are higher than than BOD. |
| 6 | BOD measurements takes 5 days | COD measurements takes 2 hrs only. |
(ii) Viable and non-viable particulate pollutants
| No | Viable particulates | Non - Viable Particulates |
| 1 | These are small sized living organisms which are dispersed in air. Eg : bacteria, fungi, moulds, algae, etc. |
These are small solid particles and liquid droplets suspended in air. Eg : Smoke, Dust, Mists, Fumes, etc. |
| 2 | Fungi causes allergy in humans and diseases in Plants. | Causes long cancer, asthma, affects mattuation of RBC, affects Photosynthesis, etc. |
| 3 | They do not help in the transportation of Particulates. | They help in transportation of viable particulates |
(i) In metal displacement reactions, we learnt that zinc replaces copper from copper sulphate solution. Let us examine whether .the reverse reaction takes place or not. As discussed earlier, place a metallic copper strip in zinc sulphate solution. If copper replaces zinc from zinc sulphate solution, Cu2+ ions would be released into the solution and the colour of the solution would change to blue. But no such change is observed. Therefore, we conclude that among zinc and copper, zinc has more tendency to release electrons and copper to accept the electrons.

(ii) Let us extend the reaction to copper metal and silver nitrate solution. Place a strip of metallic copper in sliver nitrate solution taken in a beaker. After some time, the solution slowly turns blue. This is due to the formation of' Cu2+ ions, i.e. copper replaces silver from silver nitrate. The reaction is
(iii) It indicates that between copper and silver, copper has the tendency to release electrons and silver to accept electrons.
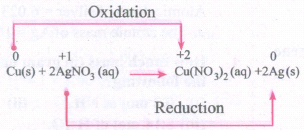
(iv) From the above experimental observations, we can conclude that among the three metals, namely, zinc, copper and silver, the electron releasing tendency is in the following order
Zinc> Copper> Silver.
Step-1: To find atoms undergoing change in O.N
\(\overset { 0 }{ P } +\overset { +1\quad +5 }{ HNO_{ 3 } } \rightarrow \overset { +1+5-2 }{ HPO_{ 3 } } +\overset { +1-2 }{ NO } +\overset { +1-2 }{ { H }_{ 2 }O } \)
Step-2: To find total decrease and increase in O.N.
P ⟶ HPO3 (increase in O.N. of 5 units per atom)
HNO3 ⟶ NO (decrease in O.N. of3 units per atom)
Total decrease 5 x 3 = 15
Total increase 3 x 5 = 15
Step-3: To balance the total increase and decrease in the equation, by multiplying P by 3 and HNO3 by 5.
3P + 5HNO3 ⟶ HPO3 + NO + H2O
Step-4: To balance all atoms other than 'O' and 'H'
3P + 5HNO3 ⟶ 3HPO3 + 5NO + H2O
Step-5: To balance by oxygen atoms
Oxygen and hydrogen atoms balance by themselves.
Hence the balanced equation is 3P + 5HNO3 ⟶ 3HPO3 + 5 NO + H2O
Heavy water is chemically similar to ordinary water (H20). However D20 reacts more slowly than H20 in chemical reactions.
(i) When compounds containing hydrogen are treated with D20, hydrogen undergoes an exchange for deuterium
2NaOH + D20 \(\rightarrow\) 2NaOD + HOD
HCI + D20\(\rightarrow\) DCI + HOD
NH4CI + 4D20 \(\rightarrow\) ND4CI + 4HOD .
These exchange reactions are useful in determining the number of ionic hydrogens present in a given compound.
For example, when D20 is treated with of hypo-phosphorus acid only one hydrogen atom is exchanged with deuterium. It indicates that, it is a monobasic acid.
H3P02 + D20 \(\rightarrow\) H2DP02 + HDO
(ii) It is also used to prepare some deuterium compounds:
Al4C3 + 12D20 \(\rightarrow\) 4AI(OD)3 + 3CD4
CaC2 + 2 D20\(\rightarrow\) Ca(OD)2 + C2D2
Mg3N2 + 6D20 \(\rightarrow\) 3Mg(OD)2 + 2 ND3
Ca3P 2+ 6D20\(\rightarrow\) 3Ca(OD)2 +2PD3
(i) Quick lime :
Preparation :
It is produced on a commercial scale by heating limestone in a lime kiln at 1173K (1070-1270).
\(CaCO_3\leftrightharpoons CaO + CO_2\)
Uses: Calcium oxide is used
(i) to manufacture cement, mortar and glass.
(ii) in the manufacture of sodium carbonate and slaked lime.
(iii) in the purification of sugar.
(iv) as drying agent
(ii) Slaked lime :
Preparation: Calcium hydroxide is prepared by adding water to quick lime, CaO.
CaO + H2O ⟶ Ca(OH)2
Uses: Calcium hydroxide is used
(I) in the preparation of mortar, a building material.
(ii) in white wash due to its disinfectant nature.
(iii) in glass making, in tanning industry, for the preparation of bleaching powder and for purification of sugar
Robert Boyle performed a series of experiments to study the relation between the Pressure and volume of gases. The schematic of the apparatus used by him is shown in figure.
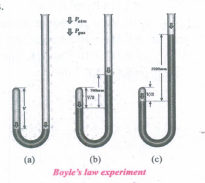
Mercury was added through the open end of the apparatus such that the mercury level on both ends are equal as shown in the figure. Add more amount of mercury until the volume of the trapped air is reduced to half of its original volume as shown in figure. The pressure exerted on the gas by the addition of excess mercury is given by the diflerence in mercury levels of the tube. Initially the pressure exerted by the gas is equal to 1 atm asthe difference in mercurylevels to 760 mm. Now the pressure exerted by the gas is equal to 2 atm. It led him to conclude that at a given temperature the volume occupied by fixed mass of a gas is inversely proportional to its Pressure.
Mathematically, the Boyle's law can be written as
\(V \alpha{{l}\over{P}}\) ....(1)
(T and n are fixed, T-temperature, n- number of moles)
\(V=k\times{{1}\over{P}}\) .....(2)
k - proportionality constant
When we rearrange equation (2)
PV = k at constant temperature and mass
Therefore, for a given mass of a gas under two different sets of conditions at constant temperature we can write
P1V1 = P2V2 = k ..............(3)
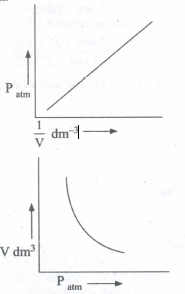
Graphical representation of Boyle's law
Given data P1 = 1 atm V1 = 5.82 dm3
P1 = 1.92 atm V2 = ?
According to Boyles law,
P1V1 = P2V2
\({ V }_{ 2 }=\frac { { P }_{ 1 }{ V }_{ 1 } }{ { P }_{ 2 } } \)
\({ V }_{ 2 }=\frac { 1\ atm\times 5.82\ { dm }^{ 3 } }{ 1.92\ atm } \)
V2 = 3.031 dm2
At sea level pressure P1 = 1 atm
Volume occupied at sea level V1 = 785 \(\times\) 10-3 dm3
If the pressure P2 = 0.052 atm
the volume of the balloon V2 = ?
According to Boyle's law
P1 V1 = P2 V2
1 \(\times\) 785 \(\times\) 10-3 = 0.052 \(\times\) V2
\({ V }_{ 2 }=\frac { 1\times 785\times { 10 }^{ -3 } }{ 0.052 } \)
= 15096.15 \(\times\) 10-3 dm3
Given:
Tc = 33.2oC + 273 = 306.2 K
PC = 12.4 atm, R = 0.082 atm lit K-1 mol-1
Sol:
\({ T }_{ C }=\frac { 8a }{ 27Rb }\)...(1)
\({ P }_{ C }=\frac { a }{ { 27 }b^{ 2 } } \)...(2)
(1) + (2)
\(\frac { { T }_{ C } }{ { P }_{ C } } =\frac { 8a }{ 27Rb } \times \frac { { 27 }b^{ 2 } }{ a } =\frac { 8b }{ R } \)
\(\therefore \frac { 306.2 }{ 12.4 } =\frac { 8\times b }{ 0.082 } \)
\(b=\frac { 306.2\times 0.082 }{ 12.4\times 8 } =0.253\)
b = 0.253 lit mol-1
Sub 'b' in (1)
\({ T }_{ C }=\frac { 8a }{ 27Rb } ;306.2=\frac { 8\times a }{ 27\times 0.082\times 0.253 } \)
\(a=\frac { 306.2\times 27\times 0.082\times 0.253 }{ 8 } =21.439\)
a = 21.439 atm lit2 mol-1
The isotherms of carbon dioxide at different temperatures which is shown.in figure.
From the plots we can infer the following:
At low temperature isotherms, for example, at 13°C as the pressure increases, the volume decreases along AB and is a gas until the point B is reached. At B, a liquid separates along the line BC, both the liquid and gas co-exist and the pressure remains constant. At C, the gas is completely converted into liquid. If the pressure is higher than at C, only the liquid is compressed so, there is no significant change in the volume. The successive isotherms shows similar trend with the shorter flat region. i.e. The volume range in which the liquid and gas coexist becomes shorter. At the temperature of 31.1°C the length of the shorter portion is reduced to zero at point P. In other words, the CO2 gas is liquefied completely at this point. This temperature is known as the liquefaction temperature or critical temperature of CO2, At this point the pressure is 73 atm. Above this temperature CO2 remains as a gas at all pressure values. It is then proved that many real gases behave in a similar manner to carbon dioxide.
q = 957.7 kJ
T = 110+273 = 383 K
ΔS= \(\frac { { q }_{ rev } }{ T } \)
ΔS = \(\frac { 957.7 }{ 383 } \)
ΔS =2.5 kJ K-1











