Use of screw gauge in measuring radius of a thin wire in the range of 10^-5 m
The wire whose diameter is to be determined should be clamped between the jaws of the screw gauge. The reading on pitch scale (P.S.R) is noted. Then the reading of the reading of the head scale coinciding with the pitch scale is noted (A.S.C.) The zero correction is applied to head scale incidence. (C.H.S.S.).
The total reading is given by
T.R = P.S.R + (C.H.S.C. X L.C)
The procedure is repeated for atleast six different positions of the wire. The mean of the reading taken gives the diameter of the wire. Half of this gives radius of the wire 'r' in the range of 10^-5 m.
Use of vernier caliper in measuring smaller distances in the range of 10^-4 m
The sphere is kept between the two jars. The main scale reading (MSR) is noted (i.e), the main scale division immediately before the zero of the vernier scale. Then the vernier scale division which coincides with some main scale division (VSD) is noted. Zero correction made with this VSD gives VSR. Multiply this VSR by least count and add with MSR. This will give the diameter of the sphere. Observations for different positions of the sphere is hence forth recorded. The mean of the readings taken gives the diameter of the sphere. Half of this gives the diameter of the sphere. Half of this gives radius in the range of 10^-4 am
(ii) Write a note on triangulation method and radar method to measure larger distances.
Triangulation method for the height of an accessible object
Let AB = h be the height of the tree or tower to be measured. Let C be the point of observation at distance x from B. Place a range finder at C and measure the angle of elevation, ∠ACB = θ as shown in Figure

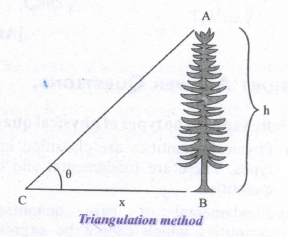
From right angled triangle ABC,
\(\tan \theta=\frac{A B}{B C}=\frac{h}{x}\)
(or) height h = x tan θ
Knowing the distance x, the height h can be determined.
Radar Method: In Radar method radio waves are sent from transmitters which, after reflection from the planet, are detected by the receiver. By measuring, the time interval (r) between the instants the radio waves are sent and received, the distance of the planet can be determined as to get the actual distance of the object. This method can also be used to determine the height, at which an aeroplane flies from the ground.
\(Speed =\frac{\text { Distance travelled }}{\text { Time taken }} \) (Speed is explained in unit 2 )
Distance (d)= Speed of radio waves x Time taken
\(d=\frac{v \times t}{2}\)
where v is the speed of the radio wave. As the time taken (r) is for the distance covered during the forward and backward path of the radio waves, it is divided by 2 to get the actual distance of the object. This method can also be used to determine the height, at which an aeroplane flies from the ground.

Consider an object thrown with initial velocity \(\overrightarrow{u}\) at an angle \(\theta\) with the horizontal.
Then,
\(\overrightarrow{u}=u,\overrightarrow{i}+u,\overrightarrow{j}\)
where u_x =u cos \(\theta\) is the horizontal component and u_y = u sin \(\theta\) the vertical component of velocity.

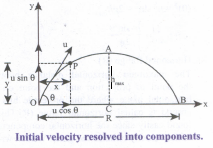
Since the acceleration due to gravity acts in the direction opposite to the direction of vertical component u_y, this component will gradually reduce to zero at the maximum height of the projectile. At this maximum height, the same gravitational force will push the projectile to move downward and fall to the ground.
But, there is no acceleration along the x direction throughout the motion. So, the horizontal component of velocity (u_x = u cos \(\theta\)) remains the same till the object reaches the ground.
After anytime t, the velocity along horizontal motion
v_x = u_x + a_xt = u_x = u cos\(\theta\)  [∴ a_x = 0]
The horizontal distance travelled by projectile in time t is
s_x = \({u}_{x}t+{{1}\over{2}}a_n{t}^{2}\)
[∴ s_x = x and a_n = 0]
∴ t = \(\frac{x}{u \cos \theta} \) ......(1)
For the vertical motion the velocity after time t is v_y = u_y + a_yt
v_y = u sin \(\theta\) - gt........(2)
(∴ u_g = u sin \(\theta\) and a_y = - g)
The vertical distance travelled by the projectile in the same time r is
s_y = \({u}_{y}t{{1}\over{2}}{a}_{y}{t}^{2}\)
y = sin \(\theta\ t-{{1}\over{2}}{gt}^{2}\)
(Here, (Here, s_y = y, u_y = u sin \(\theta\) and a_y = - g) 
Substitute the value of t from equation (1) in equation (3), we have
\(y=u\ \sin\theta{{x}\over{u\ \cos\ \theta}}-{{1}\over{2}}g{{x^2}\over{u^2{cos}^{2}\theta}}\)
\(y=x\ \tan\theta-{{1}\over{2}}g{{x^2}\over{u^2{cos}^{1}\theta}}\)
Thus the path followed by the projectile is an inverted parabola.
2. Maximum height: The maximum vertical distance travelled by the projectile during its journey is called maximum height. 
For the vertical part of the motion. \({v}_{y}^{2}={u}_{y}^{2}+2{a}_{y}s\)
Here, u_y = u sin \(\theta\),  a_y = -g, S = h_max and at the maximum height v_y = 0.
Hence,
∴ 0 = u² sin² = 2gh_max
\({h}_{max}={{{u}^{2}{sin}^{2}\theta}\over{2g}}\)
3. Horizontal Range (R): The maximum horizontal distance between the point of projection and the point on the horizontal plane where the projectile hits the ground is called horizontal range (R). 
Range R = Horizontal component of velocity x time of flight
= u cos \(\theta\times{T}_{f}\)
\(R=u\ \cos\theta\times{{2u\ \sin\theta}\over{g}}={{2{u}^{2}\sin\theta\cos\theta}\over{}g}\)      \([\therefore T_f=\frac {2u\ sin \theta}{g}]\)
\(\therefore\ R={{u^2\sin 2\theta}\over{g}}\)

Consider an inclined plane on which an object is placed as shown in the figure, Let the angle which this plane makes with the horizontal be ፀ. For small angle of ፀ, the object may not slide down. As ፀ is increased, for a particular value of ፀ, the object begins to slide down. This value is called angle of repose. Hence, the angle of repose is the angle of the inclined plane with the horizontal such that an object placed on it begins to slide.

Work-Kinetic Energy Theorem
Work and energy are equivalents. This is true in the case of kinetic energy also. To prove this, let us consider a body of mass m at rest on a frictionless horizontal surface.
The work (W) done by the constant force (F) for a displacement (s) in the same direction is,
W = Fs
The constant force is given by the equation,
F = ma
The third equation of motion can be written as,
\(v^{2} =u^{2}+2 a s  \)
\(a =\frac{v^{2}-u^{2}}{2 s}\)
Substituting for a in equation (2),
\(F=m\left(\frac{v^{2}-u^{2}}{2 s}\right)\)
Substituting equation (2), (1)
\(w=m\left(\frac{v^{2}}{2 s} s\right)-m\left(\frac{u^{2}}{2 s} s\right)  \)
\(w=\frac{1}{2} m v^{2}-\frac{1}{2} m u^{2}\)
The expression for kinetic energy:
The term \(\left(\frac{1}{2} m v^{2}\right)\) in the above equation is the kinetic energy of the body of mass (m) moving with velocity(v).
\(K E=\frac{1}{2} m v^{2}\)
Kinetic energy of the body is always positive. From equations (4) and (5)
\(\Delta K E =\frac{1}{2} m v^{2}-\frac{1}{2} m u^{2}  \)
\(\text {Thus, } W =\Delta K E\)
The expression on the right hand side (RHS) of equation (6) is the change in kinetic energy (\(\Delta\)KE) of the body.
This implies that the work done by the force on the body changes the kinetic energy of the body, This is called work-kinetic energy theorem.
The work-kinetic energy theorem implies the following.
1. If the work done by the force on the body is positive then its kinetic energy increases.
2. If the work done by the force on the body is negative then its kinetic energy decreases.
3. If there is no work done by the force on the body then there is no change in its kinetic energy, which means that the body has moved at constant speed provided its mass remains constant.

If we suspend the lamina from different points like P, Q, R as shown in Figure, the vertical lines PP', QQ', RR' all pass through the centre of gravity Here, reaction force acting at the point of suspension and the gravitational force acting at the centre of gravity cancel each other and the torques caused by them also cancel each other.

Perpendicular axis theorem:
(i) The theorem states that the moment of inertia of a plane laminar body about an axis perpendicular to its plane is equal to the sum of moments of inertia about two perpendicular axes lying in the plane of the body such that all the three axes are mutually perpendicular and have a common point.
(ii) Let the X and Y-axes lie in the plane arid Z-axis perpendicular to the plane of the laminar object. If the moments of inertia of the body about X and Y-axes are Ix and Iy respectively and It is the moment of inertia about Z-axis, then the perpendicular axis theorem could be expressed as,
 I_z = I_x + I_y
 (iii) To prove this theorem, let us consider a plane laminar object of negligible thickness on which lies the origin (0). The X and Y-axes lie on the plane and Z-axis is perpendicular to it as shown in Figure. The lamina is considered to be made up of a large number of particles of mass m. Let us choose one such particle at a point P which has coordinates (x, y) at a distance r from O.

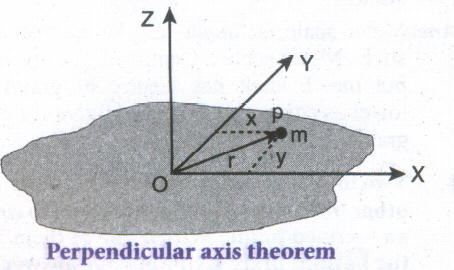
(iv) The moment of inertia of the particle about Z axis is mr². The summation of the above expression gives the moment of inertia of the entire lamina about Z-axis as, I_z = \(\Sigma \)mr²
Here r² = x² + y²
Then, I_z = \(\Sigma \)(x² +y²)
I_z = \(\Sigma \)mx² + \(\Sigma \)my²
(v) In the above expression, the term \(\Sigma \)mx² is the moment of inertia of the body about the Y-axis and similarly the term \(\Sigma \)my² is the moment of inertia about X-axis. Thus,
I_x= \(\Sigma \)my²and I_y= \(\Sigma \)mx²
Substituting in the equation for I_z gives,
I_z = I_x + I_y
Y-axis and similarly the term \(\Sigma \)my² is the moment of inertia about X-axis. Thus,
I_X = \(\Sigma \)my²and I_y= \(\Sigma \)mx²
Substituting in the equation for I_z gives,
I_z = I_x + I_y
Thus, the perpendicular axis theorem is proved.

Newton's inverse square Law:
Newton considered the orbits of the planets as circular. For circular orbit of radius r,' the centripetal acceleration towards the center is
\(a=\frac { { V }^{ 2 } }{ r } \) ...(1)

Here v is the velocity and r, the distance of the planet from the center of the orbit.
The velocity in terms of known quantities r and T, is
v = \(\frac { 2\pi r }{ T } \)    ...(2)
Here T is the time period of revolution of the planet. Substituting this value of v in equation we get,
a = \(\frac { \left( \frac { 2\pi }{ T } \right) ^{ 2 } }{ r } =-\frac { 4\pi ^{ 2 }t }{ { T }^{ 2 } } \)   ...(3)
Substituting the value of 'a' from (3) in Newton's second law, F = ma, where 'm' is the mass of the planet
F = \(\frac { 4\pi mr }{ { T }^{ 2 } } \)   ...(4)
From Kepler's third law
 \(\frac { r^{ 3 } }{ { T }^{ 2 } } \) = k(constant)   ...(5)
\(\frac { r }{ { T }^{ 2 } } =\frac { k }{ { r }^{ 2 } } \)   ....(6)
By substituting equation (6) in the force expression, we can arrive at the law of gravitation
\(F=\frac { 4\pi ^{ 2 }mk }{ { r }^{ 2 } } \) ...(7)
Here negative sign implies that the force is attractive arid it acts towards the center. In equation (7), mass of the planet 'm'. comes explicitly. But Newton strongly felt that according to his third law, if Earth is attracted by the Sun, then the Sun must also be attracted by the Earth with the same magnitude of force. So he felt that the Sun's mass (M) should also occur explicitly in the expression for force. From this insight, he equated the constant 4π²k to GM which turned out to be the law of gravitation
F = \(-\frac { GMm }{ { r }^{ 2 } } \)
Again the negative sign in the above equation implies that the gravitational force is attractive.

By finding the apparent radii of the Earth's umbra shadow and the Moon, the ratio of the these radii can be calculated. This is shown in Figure.

The apparent radius of Earth's umbra shadow
\(=\mathrm{R}_{\mathrm{s}}=13.2 \mathrm{~cm}\)
The apparent radius of the Moon
\(=\mathrm{R}_{\mathrm{m}}=5.15 \mathrm{~cm}\)
The ratio
\(\frac{R_{S}}{R_{m}} \approx 2.56\)
The radius of the Earth's umbra shadow is
\(\mathrm{R}_{\mathrm{s}}=2.56 \times \mathrm{Rm}\)
The radius of Moon \(\mathrm{R}_{\mathrm{m}}=1737 \mathrm{~km}\)
The radius of the Earth's umbra shadow is
\(\mathrm{R}_{\mathrm{s}}=2.56 \times 1737 \mathrm{~km} \cong 4446 \mathrm{~km} \text {. }\)
The correct radius is 4610 km.
The percentage of error in the calculation

There are three types of elastic modulus.
(a) Young's modulus
(b) Rigidity modulus (or Shear modulus)
(c) Bulk modulus
(a) Young's modulus:
When a wire is stretched or compressed, then the ratio between tensile stress (or compressive stress) and tensile strain (or compressive strain) is defined as Young's modulus. Young modulus of a material =\(\frac{Tensile \ stress \ or \ compressive \ stress}{Tensile \ strain\ or\ compressive\ strain}\)
\(Y=\frac{\sigma_{t}}{\epsilon_{t}} \ or \ Y=\frac{\sigma_{c}}{\epsilon_{c}}\)
The unit for Young modulus has the same unit of stress because, strain has no unit. So, S.I. unit of Young modulus is Nm^-2 or pascal.
(b) Bulk modulus:
Bulk modulus is defined as the ratio of volume stress to the volume strain.
Bulk modulus, K = \(\frac{Normal\ (perpendicular)\ stress\ or\ pressure}{Volume \ strain}\)
The normal stress or pressure is
\(\sigma_{n}=\frac{F_{n}}{\Delta A}=\Delta p\)
The volume strain is \(\epsilon_{v}= \frac{\Delta V}{V}\)
Therefore, Bulk modulus is
\(K= - \frac{\sigma_{n}}{\epsilon_{v}}= - \frac{\Delta p}{\frac{\Delta V}{V}}\)
The negative sign in the equation means that when pressure is applied on the body, its volume decreases. Further, the equation implies that a material can be easily compressed if it has a small value of bulk modulus. In other words, bulk modulus measures the resistance of solids to change in their volume.
(c) The rigidity modulus or shear modulus:
The rigidity modulus is defined as Rigidity modulus or Shear modulus,
\(\eta_{R}=\frac{shearing \ stress}{angle\ of \ shear \ or \ shearing \ strain}\)
The shearing stress is \(\sigma _{s}=\frac{trangential \ force}{area\ over\ which\ it\ is\ applied}=\frac{F_{t}}{\Delta A}\)
The angle of shear or shearing strain
\(\epsilon_{s}=\frac{x}{h}=\theta\)
Therefore, Rigidity modulus is
\(\eta = \frac{\sigma_{s}}{\epsilon_{s}}=\frac{\frac{F_{t}}{\Delta A}}{\frac{x}{h}}=\frac{\frac{F_{t}}{\Delta A}}{\theta}\)
Further, the equation implies, that a material can be easily twisted if it has small value of rigidity modulus.

(1) Excess of pressure inside air bubble in a liquid.
Consider an air bubble of radius R inside a liquid having surface tension T as shown in Figure. Let P₁ and P₂ be the pressures outside and inside the air bubble, respectively. Now, the, excess pressure inside the air bubble is
\(\Delta P=P_{1}-P_{2}.\)

In order to find the excess pressure inside the air bubble, let us consider the forces acting on the air bubble. For the hemispherical portion of the bubble, considering the forces acting on it, we get,
(i) The force due to surface tension acting towards right around the rim of length \(2 \pi \mathrm{R} \ is \ \mathrm{F}_{\mathrm{T}}=2 \pi \mathrm{RT}\)
(ii) The force due to outside pressure P₁ is to the right acting across a cross sectional area of \(\pi \mathrm{R}^{2} \  is \ F_{P_{1}}=P_{1} \pi R^{2}\)
(iii) The force due to pressure P₂ inside the bubble, acting to the left is \(F_{P_{2}}=P_{2} \pi R^{2}\).
As the air bubble is in equilibrium under the action of these forces, \(F_{P_{2}}=F_{T}+F_{P_{1}}\)
\(\mathrm{P}_{2} \pi \mathrm{R}^{2} =2 \pi \mathrm{RT}+\mathrm{P}_{1} \pi \mathrm{R}^{2}  \)
\(\Rightarrow\left(\mathrm{P}_{2}-\mathrm{P}_{1}\right) \pi \mathrm{R}^{2} =2 \pi \mathrm{RT}\)
Excess pressure is \(\Delta \mathrm{P}=P_{2}-P_{1}=\frac{2 T}{R}\)
(2) Excess pressure inside a soap bubble
Consider a soap bubble of radius R and the surface tension of the soap bubble be T. A soap bubble has two liquid surfaces in contact with air, one inside the bubble and other outside the bubble. Hence, the force on the soap bubble due to surface tension is \(2 \times 2 \pi\) RT. The various forces acting on the soap bubble are,
(i) Force due to surface tension \(F_{T}=4 \pi R T\) towards right
(ii) Force due to outside pressure, \(F_{P_{1}}=P_{1} \pi R^{2}\) towards right
(iii) Force due to inside pressure, \(F_{P_{2}}=P_{2} \pi R^{2}\) towards left
As the bubble is in equilibrium, \(F_{P_{2}}=F_{T}+F_{P_{1}}\)
\(\mathrm{P}_{2} \pi \mathrm{R}^{2} =4 \pi \mathrm{RT}+\mathrm{P}_{1} \pi \mathrm{R}^{2}  \)
\(\Rightarrow\left(\mathrm{P}_{2}-\mathrm{P}_{1}\right) =\pi \mathrm{R}^{2}=4 \pi \mathrm{RT} \pi \mathrm{R}^{2}  \)
\(\text {Excess pressure is } \Delta \mathrm{P} =\mathrm{P}_{2}-\mathrm{P}_{1}=\frac{4 T}{R}\)
(3) Excess pressure inside the liquid drop
Consider a liquid drop of radius R and the surface tension of the liquid is T. 
The various forces acting on the liquid drop are,
(i) Force due to surface tension \(F_{T}=2 \pi R T\) towards right
(ii) Force due to outside pressure, \(F_{P_{1}}=P_{1} \pi R^{2}\) towards right
(iii) Force due to inside pressure, \(F_{P_{2}}=P_{2} \pi R^{2}\) towards left
As the bubble is in equilibrium, \(F_{P_{2}}=F_{T}+F_{P_{1}}\)
\(\mathrm{P}_{2} \pi \mathrm{R}^{2} =2 \pi \mathrm{RT}+\mathrm{P}_{1} \pi \mathrm{R}^{2}  \)
\(\Rightarrow\left(\mathrm{P}_{2}-\mathrm{P}_{1}\right) \pi \mathrm{R}^{2}=2 \pi \mathrm{RT} \)
\(\text {Excess pressure is } \Delta \mathrm{P} =\mathrm{P}_{2}-\mathrm{P}_{1}=\frac{2 T}{R}\)

Meaning of heat:
When an object at higher temperature is placed in contact with another object at lower temperature, there will be a spontaneous flow of energy from the object at higher temperature to the one at lower temperature. This energy is called heat. This process of energy transfer from higher temperature object to lower temperature object is called heating. Due to flow of heat sometimes the temperature of the body will increase or sometimes it may not increase.
Examples:
(i) When it rains, lake receives water from the cloud. Once the rain stops, the lake will have more water than before raining. Here 'raining' is a process which brings water from the cloud. Rain is not a quantity rather it is water in transit. So the statement lake has more rain is wrong, instead the 'lake has more water' will be appropriate.
(ii) When heated, a cup of coffee receives heat from the stove. Once the coffee is taken from the stove, the cup of coffee has more internal energy than before. 'Heat' is the energy in transit and which flows from an object at higher temperature to an object at lower temperature. Heat is not a quantity. So the statement A hot cup of coffee has more heat' is wrong, instead 'coffee is hot' will be appropriate.
Meaning of work
When you rub your hands against each other the temperature of the hands increases. You have done some work on your hands by rubbing. The temperature of the hands increases due to this work. Now if you place your hands on the chin, the temperature of the chin increases. This is because the hands are at higher temperature than the chin. In the above example, the temperature of hands is increased due to work and temperature of the chin is increased due to heat transfer from the hands to the chin. It is shown in Figure.

By doing work on the system, the temperature in the system will increase and sometimes may not. Like heat, work is also not a quantity and through the work energy is transferred to the system. So we cannot use the word 'the object contains more work' or 'less work'.
Either the system can transfer energy to the surrounding by doing work on surrounding or the surrounding may transfer energy to the system by doing work on the system. For the transfer of energy from one body to another body through the process of work, they need not be at different temperatures.

Consider μ mole of an ideal gas in a container with volume V, pressure P and temperature T. When the gas is heated at constant volume. the temperature increases by dT. As no work is done by the gas, the heat that flows into the system will increase only the internal energy. Let the change in internal energy be dU.
If C_v is the molar specific heat capacity at constant volume.
\(\mathrm{C}_{\mathrm{v}}=\frac{1}{\mu} \frac{d U}{d T}\)
\(dU=\mu { C }_{ v }dT\)   ...(1)
Suppose the gas is heated at constant pressure so that the temperature increases by dT. If 'Q' is the heat supplied in this process and 'dV' the change in volume of the gas
\(Q=\mu { C }_{ p }dT\)......(2)
If W is the workdone by the gas in this process, then
W = PdV     .......(3)
But from the first law of thermodynamics,
Q = dU + W   ........(4)
Substituting equations (1), (2) and (3) in (4), we get,
\(\mu { C }_{ p }dT=\mu { C }_{ v }dT+PdV\) ...(5) 
For mole of ideal gas, the equation of state is given by
PV = \(\mu \)RT ~ PdV+VdP = \(\mu \)RdT ...(6)
Since the pressure is constant, dP = 0
∴ C_pdT = C_vdT+ RdT 
∴ C_p = Cv +R (or) C_p - C_v = R ...(7)
This relation is called Meyer's relation       

The specific heat of one mole of a monoatomic gas C_V = \(\frac{3}{2}\)R
For \(\mu\)₁ mole , C_V = \(\frac{3}{2}\)\(\mu\)₁R  C_p = \(\frac{5}{2}\)\(\mu\)₁ R
The specific heat of one mole of a diatomic gas
C_v = \(\frac{5}{2}\)R
For μ₂ mole, C_V = \(\frac{5}{2}\)μ₂ R C_P = \(\frac{7}{2}\)μ₂ R
The specific heat of the mixture at constant volume C_V = \(\frac{3}{2}\)\(\mu\)₁R +\(\frac{5}{2}\)\(\mu\)₂ R
The specific heat of the mixture at constant pressure C_P = \(\frac{5}{2}\)\(\mu\)₁ R + = \(\frac{7}{2}\)\(\mu\)₂ R
The adiabatic exponent \(\gamma =\frac { { C }_{ p } }{ { C }_{ V } } =\frac { 5{ \mu }_{ 1 }+{ 7\mu }_{ 2 } }{ 3{ \mu }_{ 1 }+{ 5\mu }_{ 2 } } \)

Types of Oscillation:
Free oscillations
When the oscillator is allowed to oscillate by displacing its position from equilibrium position, it oscillates with a frequency which is equal to the natural frequency of the oscillator. Such an oscillation or vibration is known as free oscillation or free vibration. In this case, the amplitude, frequency and the energy of the vibrating object remains constant.
Examples:
(i) Vibration of a tuning fork.
(ii) Vibration in a stretched string.
(iii) Oscillation of a simple pendulum
(iv) Oscillations of a spring-mass system.
Damped oscillations
(i) During the oscillation of a simple pendulum (in previous case), we have assumed that the amplitude of the oscillation is constant and also the total energy of the oscillator is constant.
(ii) But in reality, in a medium, due to the presence of friction and air drag, the amplitude of oscillation decreases as time progresses.
(iii) It implies that the oscillation is not sustained and the energy of the SHM decreases gradually indicating the loss of energy.
(iv) The energy lost is absorbed by the surrounding medium. This type of oscillatory motion is known as damped oscillation.
(v) If an oscillator moves in a resistive medium, its amplitude goes on decreasing and the energy of the oscillator is used to do work against the resistive medium.
(vi) The motion of the oscillator is said to be damped and in this case, the resistive force (or damping force) is proportional to the velocity of the oscillator.

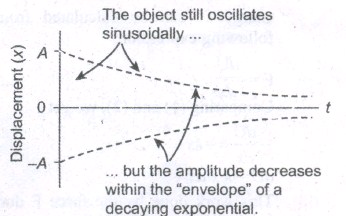
Examples:
(i) The oscillations of a pendulum (including air friction) or pendulum oscillating inside an oil filled container.
(ii) Electromagnetic oscillations in a tank circuit.
(iii) Oscillations in a dead beat and ballistic galvanometers.
Maintained oscillations:
(i) While playing in swing, the oscillations will stop after a few cycles, this is due to damping.
(ii) To avoid damping we have to supply a push to sustain oscillations. By supplying energy from an external source, the amplitude of the oscillation can be made constant.
(iii) Such vibrations are known as maintained vibrations.
Example:
The vibration of a tuning fork getting energy from a battery or from external power supply.
Forced oscillations:
(i) Any oscillator driven by an external periodic agency to overcome the damping is known as forced oscillator or driven oscillator.
(ii) In this type of vibration, the body executing vibration initially vibrates with its natural frequency and due to the presence of external periodic force, the body later vibrates with the frequency of the applied periodic force.
(iii) Such vibrations are known as forced vibrations.
Example:
Sound boards of stringed instruments.

 Consider an elemental segment in the string as shown in the Figure.

(a) Transverse waves in a stretched string.
(b) Elemental segment in a stretched string is zoomed and the pulse seen from an observer frame who moves with velocity v.
Let A and B be two points on the string at an instant of time. Let dl and dm be the length and mass of the elemental string, respectively. By definition, linear mass density, \(\mu\) is
\(\mu=\frac{dm}{dt}\) ......................(1)
dm = \(\mu\) dl ...................(2)
The elemental string AB has a curvature which looks like an arc of a circle with centre at 0, radius R and the arc subtending an angle \(\theta\) at the origin O as shown in Figure.

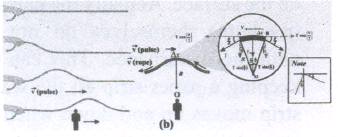
The angle e can be written in terms of arc length and radius as \(\theta=\frac{dl}{R}\). The centripetal acceleration supplied by the tension in the string is
a_cp  = \(\frac{V^2}{R}\) ................ (3)

Then, centripetal force can be obtained when mass of the string (dm) IS included in equation (3)
F_ep  = \(\frac{(dm)v^2}{R}\) ................... (4)
The centripetal force experienced by elemental string can be calculated by substituting equation (2) in equation (4) we get
\(\frac{(dm)v^2}{R}=\frac{\mu v^2\ dl}{R}\) ......................(5)
The tension T acts along the tangent of the elemental segment of the string at A and B. Since the arc length is very small, variation in the tension force can be ignored. T is resolved into horizontal component \(T\cos(\frac{\theta}{2})\) and verical component \(T\ Sin(\frac{\theta}{2})\)
The horizontal components at A and B ar equal in magnitude but opposite in direction; therefore, they cancel each other. Since the elemental arc length AB is taken to be very small, the vertical components at A and B appears to acts vertical towards the centre of the arc and hence, they add up. The net radial force F_r is
F_r = \(2T\ Sin(\frac{\theta }{2})\) ................... (6)
Since the amplitude of the wave is very small when it is compared with the length of the string, the sine of small angle is approximated as \(\ Sin(\frac{A}{2})\approx \frac{A}{2}\). Hence equation (6) can be written as
F_r = \(2T\times \frac{\theta }{2}=T\theta\) ................... (7)
But \(\theta =\frac{di}{R},\) therefore substituting in equation (7), we get
F_r = \(T\frac{dl}{R}\) .....................(8)
Applying Newton's second law to the elemental string in the radial direction, under equilibrium, the radial component of the force is equal to the centripetal force. Hence equating equation (5) and equation (8), we have
\(T\frac{dl}{R}=\mu v^2\frac{di}{R}\)
\(V=\sqrt{\frac{T}{\mu}}\) measured in ms^-1 .............(9)

(i) Physics is the most fundamental branch of science. It has played a key role in the development of all branches of sciences.
Physics in relation to mathematics
(i) Physics is a quantitative science. Mathematics provides the necessary signs and tools which the physicist use.
(ii) It has played an important role in the development of theoretical physics.
(iii) Had newton not invented calculus, he would not have been able to discover the universal law of gravitation.
Physics in relation to chemistry:
(i) In physics, we study the structure of atom, radioactivity, X-ray diffraction, etc.
(ii) Such studies have enabled chemists to arrange elements in the periodic table on the basis of their atomic numbers.
(iii) This has further helped to know the nature of valency and chemical bonding and to understand the complex chemical structures.
Physics in relation to biology.
(i) The developments in life sciences a great deal to physics.
(ii) Optical microscopes are extensively used in the study of biology.
(iii) With the help of an electron microscope, one can study the structure of cell.
(iv) The X-rays and neutron diffraction techniques have helped in understanding the structure of nucleic acids, which helped to control vital life process.
(v) Radio isotopes are used in radiation therapy for the cure of deadly diseases like cancer.

Dimension for distance s = [L]
Dimension for initial velocity v= [LT^-1]
Dimension for time t = [T]
Dimension for acceleration a = [LT^-2]
According to the principle of homogeneity,
Dimensions of LHS = Dimensions of RHS
Substituting the dimensions in the given formula
S = ut + 1/4 at², \(\frac{1}{4}\) is a number. It has no dimension
[L] = [LT^-1][T¹] + [LT^-2][T²]
[L] = [L] + [L]
As the dimensional formula of LHS is same as that of RHS, the equation is dimensionally correct.
Comment:
But actually it is a wrong equation. We know that the equation of motion is
s = ut +1/2 at²
So, a dimensionally correct equation need not be the true (or) actual equation. But a true equation is always dimensionally correct.

(i) Consider an object moving along a circle of radius r. In a time \(\Delta \) t, the object travels an arc distance \(\Delta\)s as shown in Figure. The corresponding angle subtended is \(\Delta\) \(\theta\) .

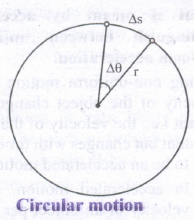
(ii) The \(\Delta\)s can be written in terms of \(\Delta\)\(\theta\) as,
\(\Delta\)s=r\(\Delta\)\(\theta\)
In a time \(\Delta\)t, we have
\(\frac { \Delta s }{ \Delta t } =r\frac { \Delta \theta }{ \Delta t } \)
(iii) In the limit \(\Delta\)t \(\rightarrow\) 0, the above equation becomes
\(\frac { ds }{ dt } =r\omega \)  .............(i)
(iv) Here \(\frac { ds }{ dt } \) is linear speed (v) which is tangential to the circle and \(\omega \) is angular speed.
(v) So equation (i) becomes v = r\(\omega \) 
(vi)This equation is true only for circular motion. In general, the relation between linear and angular velocity is given by \(\vec{v}=\vec{\omega} \times \vec{r}\)
(vii) The direction of linear velocity i is tangential to the circle, whereas the direction of angular velocity \(\vec{\omega}\) is along the axis of rotation following the right-hand rule. The radius is also represented as a vector \(\vec{r}\) directed radially from the centre of the circle.

Let \(\overrightarrow{A},\overrightarrow{B}\) and \(\overrightarrow{C}\) be represented in component from,
\(\overrightarrow{A}=\overrightarrow{A}_z\hat{i}+\hat{j}\overrightarrow{A}_y\hat{j}+\overrightarrow{A}_z\hat{k}\)
\(\overrightarrow{B}=\overrightarrow{B}_z\hat{i}+\overrightarrow{B}_y\hat{j}+\overrightarrow{B}_z\hat{k}\)
\(\overrightarrow{C}=\overrightarrow{C}_x\hat{i}+\overrightarrow{C}_y\hat{j}+\overrightarrow{C}_z\hat{k}\)
Let \(\overrightarrow{D}\) be their summation vector,
\(\overrightarrow{D}=(\overrightarrow{A}+\overrightarrow{B}+\overrightarrow{C})\)
\(=\left( (\overrightarrow{A} _x\hat{i}+\overrightarrow{A}_y\hat{j}+\overrightarrow{A}_z\hat{k})+(\overrightarrow{B}_x\hat{i}+\overrightarrow{B}_y\hat{j}+\overrightarrow{B}_z\overrightarrow{k} +\overrightarrow{C}_x\hat{i}+\overrightarrow{C}_y\hat{j}+\overrightarrow{C}_z\hat{k}\right)\)
Addition of vectors obey the commutative as well as associative laws.
\(A_y+B_y+C_yD=(A_x+B_x+C_x){2 u \sin \theta\over g}+(A_y+B_y+C_y)\hat{j}+(A_z+B_z+C_z)\hat{k}+(A_x+B_x+C_x)\hat{i}+(A_yB_y+C_y){2\ u\ \sin \theta\over g}(A_z+B_z+C_z)\hat{k}+(A_x+B_x+C_x)\hat{i}+(A_y+B_y+C_y)\hat{j}+(A_z+B_z+C_z){2\ u \sin \theta \over g}\)D_x = A_x + B_x+ C_x
D_y = A_y + B_y+ C_y
D_z = A_z + B_z + C_z

Consider the velocities \(\overrightarrow { { V }_{ A } } \)  and \(\overrightarrow { { V }_{ B } } \)  at an angle \(\theta \)  between their directions.
The relative velocity of A with respect to B,
\(\overrightarrow { { V }_{ AB } } =-\overrightarrow { { V }_{ B } } -\overrightarrow { { V }_{ A } } \)
Then, the magnitude and direction of \(\overrightarrow { { V }_{ AB } } \) is given by V_AB= \({ v }_{ aB }=\sqrt { { v }_{ A }^{ 2 }+{ v }_{ B }^{ 2 }-2{ v }_{ A }{ v }_{ B }cos\theta } \) and tan \(\beta =\frac { { v }_{ B }sin\theta }{ { v }_{ B }-{ v }_{ A }cos\theta } \) (Here \(\beta\)  is angle between \(\overrightarrow { { V }_{ AB } } and\quad \overrightarrow { { V }_{ B } } \) ) 
(i) When \(\theta\)  = 0, the bodies move along parallel straight lines in the same direction, We have v_AB = (v_A - v_B) in the direction of \(\overrightarrow { { V }_{ A } } \) . Obviously v_BA= (V_B+ v_A) in the direction of \(\overrightarrow { { V }_{ B } } \).
(ii) When \(\theta\) = 180°, the bodies move along parallel straight lines in opposite directions, We have v_AB= (v_A + V_B) in the direction of \(\overrightarrow { { V }_{ A } } \) .Similarly v_BA= (V_B+ v_A) in the direction of \(\overrightarrow { { V }_{ B } } \).
(iii) If the two bodies are moving at right angles to each other, then \(\theta\) =90°. The magnitude of the relative velocity of A with respect to \(B={ v }_{ BA }=\sqrt { { v }_{ A }^{ 2 }+{ v }_{ B }^{ 2 } } \).

(i) maximum height(h_max)
The maximum vertical distance travelled by the projectile during its journey is called maximum height. This is determined as follows: For the vertical part of the motion
\(v_{y}^{2}=u_{y}^{2}+2 a_{y} s\)
Here, u_y = u sin \(\theta\), a = -g, s =h_max, and at the maximum height v_y = 0
Hence, \((0)^{2}=u^{2} \sin ^{2} \theta=2 g h_{\max }\)
\(\text { or } \mathrm{h}_{\max }=\frac{u^{2} \sin ^{2} \theta}{2 g}\)
(ii) time of flight (T_f)
The total time taken by the projectile from the point of projection till it hits the horizontal plane is called time of flight.
This time of flight is the time taken by the projectile to go from point O to B via point A.
We know that s_y = u_yt + \(\frac{1}{2}\) a_yt²
Here, s_y = y = 0(net displacement in y-direction is zero),u_y = u sin\(\theta\), a_y = -g, t = T_f
Then \(0=\mathrm{u} \sin \theta \mathrm{T}_{\mathrm{f}}-\frac{1}{2} g T_{f}^{2}\)
\(\mathrm{T}_{\mathrm{f}}=2 \mathrm{u} \frac{\sin \mathrm{\theta}}{g}\)
(iii) horizontal range
The maximum horizontal distance between the point of projection and the point on the horizontal plane where the projectile hits the ground is called horizontal range (R). This is found easily since the horizontal component of initial velocity remains the same. We can write Range
R = Horizontal component of velocity x time of flight = u cos \(\theta\) \(\times\)T_f
\(\mathrm{R}=\mathrm{u} \cos \theta \times \frac{2u \sin \theta}{g}=\frac{2 u^{2} \sin \theta \cos \theta}{g}\)
\(\therefore \mathrm{R}=\frac{u^{2} \sin 2 \theta}{g}\)
The horizontal rate directly depends on the initial speed (u) and the sine of angle of projection ( \(\theta\) ). It inversely depends on acceleration due to gravity 'g'
For a given initial speed u, the maximum possible range is reached when sin 2 \(\theta\) is maximum, sin2 \(\theta\) = 1. This implies 2\(\theta\) = \(\pi\) /2.
or \(\theta=\frac{\pi}{4}\)
This means that if the particle is projected at 45 degrees with respect to horizontal, it attains maximum range, given by.
\(R_{\max }=\frac{u^{2}}{g}\)

(i) Consider the case of a whirling motion of a stone tied to a string. Assume that the stone has angular velocity ω in the inertial frame (at rest).
(ii) If the motion of the stone is observed from a frame which is also rotating along with the stone with same angular velocity ω then, the stone appears to be at rest.
(iii) This implies that in addition to the inward centripetal force - mω²r there must be an equal and opposite force that acts on the stone outward with value + mω²r.
(iv) So the total force acting on the stone in a rotating frame is equal to zero (-mω²r + mω²r=0).
(v) This outward force + mω²r is called the centrifugal force.

The following figure shows the forces acting on the horizontal and vertical direction.
When the car takes turn with the speed v, the centripetal force is exerted by horizontal component of normal force and static frictional force. It is given by

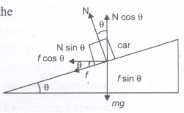
N sinθ+f cosθ=\(\frac { mv^{ 2 } }{ r } \)        ......(1)
In the vertical direction, there is no acceleration. It implies that the vertical component of normal force is balanced by downward gravitational force and downward vertical component of frictional force. This can be expressed as
N cosθ=mg+f sinθ
or N cosθ-f sinθ=mg
Diving the equation (1) by equation (2), we get
\(\frac { Nsin\theta +fcos\theta }{ Ncos\theta -fsin\theta } =\frac { { v }^{ 2 } }{ rg } \)
To calculate the maximum speed for the safe turn, we can use the maximum static friction is given by. By substituting this relation in equation (3), we get
\(\frac { Nsin\theta +{ \mu }_{ s }Ncos\theta }{ Ncos\theta -{ \mu }_{ s }Nsin\theta } =\frac { { v }_{ max }^{ 2 } }{ rg } \)
By taking outside the bracket in L.H. S of equation
\(\frac { Ncos\left\{ \left( \frac { Nsin\theta }{ Ncos\theta } \right) +{ \mu }_{ s } \right\} }{ Ncos\theta \left( 1-{ \mu }_{ s }\frac { Nsin\theta }{ Ncos\theta } \right) } =\frac { { v }_{ max }^{ 2 } }{ rg } \)
\(\frac { (tan\theta +{ \mu }_{ s }) }{ 1-{ \mu }_{ s }tan\theta } =\frac { { v }_{ max }^{ 2 } }{ rg } \)
The Maximum speed for safe turn is given. by
vmax=\(\sqrt { rg\frac { (tan\theta +{ \mu }_{ s }) }{ (1-{ \mu }_{ s }tan\theta ) } } \)
Suppose we neglect the effect of friction (μ_s = 0), then safe speed
vsafe=\(\sqrt { rgtan\theta } \)
Note that the maximum speed with which the car takes safe turn is increased by friction (equation (4)). Suppose the car turns with speed v < _-safe then the stati~ friction acts up in the slope to prevent from inward skidding.
If the car turns with the speed little greater than, then the static friction acts down the slope to prevent outward skidding. But if the car turns with the speed greater than then static friction cannot prevent from outward skidding.

\(\vec { { r }_{ 1 } } =(2\overset { \wedge }{ i } +\overset { \wedge }{ j } -\overset { \wedge }{ 3k } )\)
\(\vec { { r }_{ 2 } } =(4\overset { \wedge }{ i } +6\overset { \wedge }{ j } -7\overset { \wedge }{ k } )\)
The position vectors \(\vec{r}=\vec{r_2}-\vec{r_1}\)
=\(\left( 4\overset { \wedge }{ i } +\overset { \wedge }{ 6 } j-\overset { \wedge }{ 7k } \right) -\left( \overset { \wedge }{ 2i } +\overset { \wedge }{ j } -\overset { \wedge }{ 3k } \right) \)
\(=\overset { \wedge }{ 4i } +\overset { \wedge }{ 6j } -\overset { \wedge }{ 7k } -\overset { \wedge }{ 2i } -\overset { \wedge }{ j } +\overset { \wedge }{ 3k } \)
\(=\overset { \wedge }{ i } (4-2)+\overset { \wedge }{ j } (6-1)+\overset { \wedge }{ k } (-7+3)\)
\(=\overset { \wedge }{ i } (2)+\overset { \wedge }{ j } (5)+\overset { \wedge }{ k } (-4)\)
\(\vec { r } =\vec { { r }_{ 2 } } -\vec { { r }_{ 1 } } =2\overset { \wedge }{ i } +5\overset { \wedge }{ j } -\overset { \wedge }{ 4k } \)
The effect of force  = \(\vec { F } =(3\overset { \wedge }{ i } +2\overset { \wedge }{ j } +\overset { \wedge }{ 4k } )N\)
Workdone W = \(\vec{F}.\vec{x}\)
\(=(3\overset { \wedge }{ i } +2\overset { \wedge }{ j } +\overset { \wedge }{ 4k } ).(2\overset { \wedge }{ i } +5\overset { \wedge }{ j } -\overset { \wedge }{ 4k } )\)
\(=\overset { \wedge }{ i } .\overset { \wedge }{ j } .\overset { \wedge }{ k } (6+10-16)\)
= 1(0)
Work done = 0.

Solution:

Let the particle of mass m move with constant velocity i.  As it is moving with constant velocity its path is a straight line. Its momentum \((\overrightarrow{\mathrm{p}}=\mathrm{m} \overrightarrow{\mathrm{v}})\) is also directed along the same path. Let us fix an origin (O) at a perpendicular distance (d) from the path. At a particular instant, we can connect the particle which is at position Q with a position vector \((\vec{r}=\overline{O Q})\). Take the angle between the i and p as 0. The magnitude of angular momentum of that particle at that instant is,
\(\mathrm{L}=\mathrm{OQ} \mathrm{p} \sin \theta=\mathrm{OQ} \mathrm{mv} \sin \theta=\mathrm{mv}(\mathrm{OQ} \sin \theta)\)
The term \((O Q \sin \theta)\) is the perpendicular distance (d) between the origin and line along which the mass is moving. Hence, the angular momentum of the particte about the origin is,
L = mvd
The above expression for angular momentum L, does not have the angle 0. As the momentum (p = mv) and the perpendicular distance (d) are constants, the angular momentum of the particle is also constant. Hence, the angular momentum is associated with bodies with linear motion also. If the straight path of the particle passes through the origin, then the angular momentum is zero, which is also a constant.

\(\text {Mass of the earth } \mathrm{M} =6 \times 10^{24} \mathrm{~kg}  \)
\(\text {Radius of the earth } \mathrm{R} =6.4 \times 10^{6} \mathrm{~m}  \)
\(\text {Height of the satellite } \quad \mathrm{h} =5 \times 10^{5} \mathrm{~m}=0.5 \times 10^{6} \mathrm{~m}  \)
\(\text {Mass of the satellite } \quad \mathrm{m} =600 \mathrm{~kg}  \)
\(\text {Gravitational constant } \quad \mathrm{G} =6.67 \times 10^{-11} \mathrm{Nm}^{2} \mathrm{~kg}^{-2}  \)
\(\text {Kinetic energy } =\frac{G M m}{2(\mathrm{R}+\mathrm{h})}  \)
\(=\frac{6.67 \times 10^{-11} \times 6 \times 10^{24} \times 600}{2[6.4+0.5] \times 10^{6}}  \)
\(=\frac{6.67 \times 10^{-11} \times 36 \times 10^{26}}{2 \times 6.9 \times 10^{6}}  \)
\(=17.4 \times 10^{9} \mathrm{~J}  \)
\(K.E=1.74 \times 10^{10} \mathrm{~J}\)
Potential energy \(\mathrm{E}_{\mathrm{P}} =\frac{-G M m}{R+h}  \)
\(\mathrm{E}_{\mathrm{p}} =\frac{-6.67 \times 10^{-11} \times 6 \times 10^{24} \times 600}{6.4 \times 10^{6}+0.5 \times 10^{6}}  \)
\(=\frac{-6.67 \times 10^{-11} \times 36 \times 10^{26}}{6.9 \times 10^{6}}  \)
\(\mathrm{E}_{\mathrm{P}} =-3.48 \times 10^{10} \mathrm{~J}  \)
Total energy \(\mathrm{E}=\mathrm{E}_{\mathrm{p}}+\mathrm{E}_{\mathrm{K}}  \)
\(\mathrm{E} =-3.48 \times 10^{10}+1.74 \times 10^{10}  \)
\(\mathrm{E}_{\mathrm{T}} =1.74 \times 10^{10} \mathrm{~J}\)

Given:
Atmospheric pressure
\(P =10^{5} \mathrm{Nm}^{-2}\)
Density \(\rho=9000 \mathrm{~kg} \mathrm{~m}^{-3}\)
Specific heat \(C=400 \mathrm{~J} \mathrm{~kg}^{-10} \mathrm{C}^{-1}\)
Co-efficient of cubical expansion
\(\gamma =9 \times 10^{-50} \mathrm{C}^{-1}  \)
\(\Delta Q =m s \Delta T\)
(i) Rise in temperature \(\Delta T=\frac{\Delta Q}{m s}\)
\(\Delta T=\frac{20000}{1 \times 400} \)
\(=50^{\circ} \mathrm{C}\)
(ii) Density \(\rho=\frac{M}{V}\)
Volume \(\rho=\frac{M}{V}\) 
\(=\frac{1}{9000} m^{3}\)
Change in volume \(\Delta V=\gamma V \Delta T\)
\(=9 \times 10^{-5} \times \frac{1}{9000} \times 50  \)
\(=5 \times 10^{-7} \mathrm{~m}^{3}  \)
\(\therefore \text {Work done } W =P \Delta V  \)
\(=10^{5} \times 5 \times 10^{-7}=0.05 \mathrm{~J}\)
(iii) Change in internal energy
\(\Delta U =\Delta Q-\Delta W \)
=20000-0.05
19999.95 J

(a) The specific heat of one mole of a mono atomic.
\(\begin{equation} \operatorname{Gas} \mathrm{C}_{\mathrm{v}}=\frac{3}{2} R \end{equation}\) 
\(\begin{equation} \text { For } \mu_{1} \text { mole, } C_{v}=\frac{3}{2} \mu_{1} R \quad C_{p}=\frac{5}{2} \mu_{1} R \end{equation}\) 
The specific heat of one mole of a diatomic gas
\(\begin{equation} \mathrm{C}_{\mathrm{v}}=\frac{5}{2} R \end{equation}\) 
\(\begin{equation} \text { For } \mu_{2}, \text { mole, } C_{\mathrm{V}}=\frac{5}{2} \mu_{2} R \quad C_{P}=\frac{7}{2} \mu_{2} R \end{equation}\) 
The specific heat of the mixture at constant
\(\begin{equation} \text { Volume } \ C_{v}=\frac{3}{2} \mu_{1} R+\frac{5}{2} \mu_{2} R \end{equation}\) 
 The specific heat of the mixture at constant
\(\begin{equation} \text { Pressure } C_{\mathrm{P}}=\frac{5}{2} \mu_{1} R+\frac{7}{2} \mu_{2} R \end{equation}\) 
The adiabatic exponent \(\begin{equation} \gamma=\frac{C_{P}}{C_{v}} \end{equation}\) 
\(\begin{equation} =\frac{5 \mu_{1}+7 \mu_{2}}{3 \mu_{1}+5 \mu_{2}} \end{equation}\)
(b) \(\begin{equation} \lambda=\frac{1}{\sqrt{2} \pi n d^{2}} \end{equation}\)
we have to find the number density n by using ideal gas law.
\(\begin{equation} \mathrm{n}=\frac{N}{V}=\frac{P}{k T} \end{equation}\)
\(\begin{equation} =\frac{101.3 \times 10^{3}}{1.381 \times 10^{-23} \times 300} \end{equation}\)
= 2.449\(\times\)10²⁵ molecules/m³
\(\begin{equation} \lambda=\frac{1}{\sqrt{2} \times \pi \times 2.449 \times 10^{25} \times\left(1.2 \times 10^{-10}\right)^{2}} \end{equation}\)
\(\begin{equation} =\frac{1}{15.65 \times 10^{5}} \end{equation}\) 
\(\begin{equation} \lambda=0.63 \times 10^{-6} \mathrm{~m} \end{equation}\)

(1) Free oscillation:
(i) The oscillation of a particle with fundamental frequency under the influence of restoring force are defined as free oscillation.
(ii) The amplitude, frequency, and energy of oscillation remains constant.
(iii) Frequency of oscillation is called natural frequency because it depends upon the nature and structure of the body.

(2) Damped oscillation:
(i) The oscillation of a body whos amplitude goes on decreasing with time are defined as damped oscillation.
(ii) In these oscillation the amplitude oscillation decreases exponentially due to damping forces like frictional fore viscouse force, etc.
(iii) Due to decrease in amplitude the energy of the oscillator also goes on decreasing exponentially.

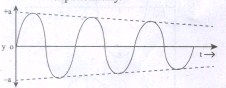
(iv) The force produces a resistance to the oscillation is called damping force.
If the velocity of oscillator is v then Damping force Fd=-bv, b = damping constant.
(v) Resultant force on a damped oscillator is given by
F = F_R+F_d= -kx-kv ⇒ \(\frac { md^{ 2 }x }{ dt^{ 2 } } +b\frac { dx }{ dt } +kx\) = 0
(vi) Displacement of damped oscillator given by
x = \({ x }_{ m }e^{ \frac { bt }{ 2m } }sin(w't+\psi )\)
where w' = angular frequency of the damped oscillator = \(\sqrt { { W }_{ 0 }^{ 2 }-\left( \frac { b }{ 2m } \right) ^{ 2 } } \)
The amplitude decreases continuously with time according to x =\(x_{ m }e^{ \left( \frac { b }{ 2m } \right) t }\)
(vii) For a damped oscillator if the damping is small then the mechanical energy decreases exponentially with time as
E = \(\frac { 1 }{ 2 } kx_{ m }^{ 2 }e^{ \frac { ht }{ m } }\) .

In air t₀=\(2\pi \sqrt { \frac { l }{ g } } \)
Let V be the volume of the bob. Then apparent weight of bob in water = weight of bob in air - up thrust
\(V\rho { g }^{ ' }=V\rho g-v\sigma g\)
\({ g }^{ ' }=\left( 1-\frac { \sigma }{ \rho } \right) g\)
Density of bob, \(\rho \)=\(\frac{400}{3}\) kg m^-3
Density of water, \(\sigma \)= 1000 kg m^-3
g^'=\(\left( 1-\frac { 1000\times 3 }{ 4000 } \right) g\)
=\(\frac{g}{4}\)
Time period of the-pendulum in water
t=\(2\pi \sqrt { \frac { l }{ g } } =2\pi \sqrt { \frac { l }{ \frac { g }{ 4 } } } =2\times 2\pi \sqrt { \frac { l }{ g } } \)