









By QB365 on 31 Dec, 2022
QB365 provides a detailed and simple solution for every Possible Questions in Class 11 Physics Subject - Revision Model Question Paper, English Medium. It will help Students to get more practice questions, Students can Practice these question papers in addition to score best marks.
11th Standard
Physics
PART-I
Note : i ) All Questions Are Compulsory.
ii) Choose The Most Suitable Answer From The Given Four Correct Alternatives.
If the error in the measurement of radius is 2%, then the error in the determination of volume of the sphere will be
8%
2%
4%
6%
A ball is projected vertically upwards with a velocity v. It comes back to ground in time t. Which v-t graph shows the motion correctly?




Choose appropriate free body diagram for the particle experiencing net acceleration along negative y direction. (Each arrow mark represents the force acting on the system).
A uniform force of (2\(\hat { i }\)+\(\hat { j }\)) N acts on a particle of mass 1 kg. The particle displaces from position (3\(\hat { j }\)+\(\hat { k }\)) m to (5\(\hat { i }\)+3\(\hat { j }\)) m. The work done by the force on the particle is
9 J
6 J
10 J
12 J
A disc of the moment of inertia Ia is rotating in a horizontal plane about its symmetry axis with a constant angular speed \(\omega\). Another disc initially at rest of moment of inertia Ib is dropped coaxially on to the rotating disc. Then, both the discs rotate with the same constant angular speed. The loss of kinetic energy due to friction in this process is,
\(\frac { 1 }{ 2 } \frac { { I }_{ b }^{ 2 } }{ 2({ I }_{ a }+{ I }_{ b }) } { \omega }^{ 2 }\)
\(\frac { { I }_{ b }^{ 2 } }{ ({ I }_{ a }+{ I }_{ b }) } { \omega }^{ 2 }\)
\(\frac { { ({ I }_{ b }-{ I }_{ a }) }^{ 2 } }{ ({ I }_{ a }+{ I }_{ b }) } { \omega }^{ 2 }\)
\(\frac { 1 }{ 2 } \frac { { { I }_{ b }{ I }_{ b } } }{ ({ I }_{ a }+{ I }_{ b }) } { \omega }^{ 2 }\)
If the mass and radius of the Earth are both doubled, then the acceleration due to gravity g'
remains same
\({g\over 2}\)
2g
4g
The load – elongation graph of three wires of the same material are shown in figure. Which of the following wire is the thickest?
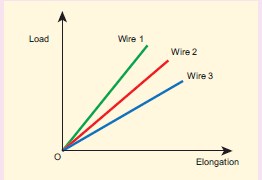
wire 1
wire 2
wire 3
all of them have same thickness
When a cycle tyre suddenly bursts, the air inside the tyre expands. This process is
isothermal
adiabatic
isobaric
isochoric
The following graph represents the pressure versus number density for ideal gas at two different temperatures T1 and T2. The graph implies

T1 = T2
T1 > T2
T1 < T2
Cannot be determined
The time period for small vertical oscillations of block of mass m when the masses of the pulleys are negligible and spring constant k1 and k2 is
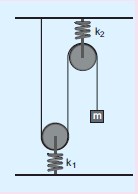
\(T=4\pi \sqrt { m\left( \frac { 1 }{ { k }_{ 1 } } +\frac { 1 }{ { k }_{ 2 } } \right) } \)
\(T=2\pi \sqrt { m\left( \frac { 1 }{ { k }_{ 1 } } +\frac { 1 }{ { k }_{ 2 } } \right) } \)
\(T=4\pi \sqrt { m\left( { k }_{ 1 }+{ k }_{ 2 } \right) } \)
\(T=2\pi \sqrt { m\left( { k }_{ 1 }+{ k }_{ 2 } \right) } \)
Which of the following options is correct?.
| A | B |
| (1) Quality | (A) Intensity |
| (2) Pitch | (B) Waveform |
| (3) Loudness | (C) Frequency |
Options for (1), (2) and (3), respectively are
(B), (C) and (A)
(C), (A) and (B)
(A), (B) and (C)
(B), (A) and (C)
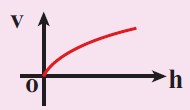
A uniform rope having mass m hangs vertically from a rigid support. A transverse wave pulse is produced at the lower end. Which of the following plots shows the correct variation of speed v with height h from the lower end?




How many light years make 1 per sec?
3.26
6.67
1.5
9.4
How many \(\mu\)m present in one metre?
10-6 \(\mu\)m
106 \(\mu\)m
10-3 \(\mu\)m
10-2 \(\mu\)m
In hot summer after a bath, the body’s
internal energy decreases
internal energy increases
heat decreases
no change in internal energy and heat
PART-II
Note : i
) Answer any Six Questions and Question.No: 24 is compulsory.
State principle of moments.
State Kepler’s three laws.
A wire 10 m long has a cross-sectional area 1.25\(\times\)10-4 m2. It is subjected to a load of 5 kg. If Young’s modulus of the material is 4\(\times\)1010 N m-2, calculate the elongation produced in the wire. Take g = 10 ms-2.
We can cut vegetables easily with a sharp knife as compared to a blunt knife. Why?
A person does 30 kJ work on 2 kg of water by stirring using a paddle wheel. While stirring, around 5 kcal of heat is released from water through its container to the surface and surroundings by thermal conduction and radiation. What is the change in internal energy of the system?
What is PV diagram?
Classify the following motions as periodic and non-periodic motions?
a. Motion of Halley’s comet.
b. Motion of clouds.
c. Moon revolving around the Earth
An increase in pressure of 100 kPa causes a certain volume of water to decrease by 0.005% of its original volume.
(a) Calculate the bulk modulus of water?
(b) Compute the speed of sound (compressional waves) in water?
Write a note an scope of physics.
PART-III
Note : i
) Answer any Six Questions and Question.No: 33 is compulsory.
A solid sphere has a radius of 1.5 cm and a mass of 0.038 kg. Calculate the specific gravity or relative density of the sphere.
When you mix a tumbler of hot water with one bucket of normal water, what will be the direction of heat flow? Justify.
What is a thermal expansion?
An oxygen molecule is travelling in air at 300 K and 1 atm, and the diameter of oxygen molecule is 1.2\(\times\)10−10m. Calculate the mean free path of oxygen molecule.
Show that for a simple harmonic motion, the phase difference between
a. displacement and velocity is \(\frac{\pi}{2}\) radian or 90°.
b. velocity and acceleration is \(\frac{\pi}{2}\) radian or 90°.
c. displacement and acceleration is \(\pi\) radian or 180°.
Explain damped oscillation. Give an example.
N tuning forks are arranged in order of increasing frequency and any two successive tuning forks give n beats per second when sounded together. If the last fork gives double the frequency of the first (called as octave), Show that the frequency of the first tuning fork is f = (N−1)n.
Differentiate fundamental quantities from derived quantities.
In a physical units, how many units are there in 1 metre?
1 Astronomical unit (AU = 1.496\(\times\)1011 m)
Given data:
1 AU = 1.496\(\times\)1011m
1 ly = 9.467 x \(\times\)1015m
1 mm = 10-6m
1 parsec = 3.08\(\times\)1016m
PART-IV
Note : i ) Write all the following questions.
Explain in detail the triangle law of addition.
State and explain work energy principle. Mention any three examples for it.
Explain in detail the Eratosthenes method of finding the radius of Earth.
Explain in detail the isochoric process.
Describe the vertical oscillations of a spring.
Explain how overtones are produced in a
(a) Closed organ pipe
(b) Open organ pipe
The value Gin CGS system is 6.67\(\times\)10-8 dyne cm2 g-2. Calculate the value in SI units.
The frequency of vibration of a string depends of on,
(i) tension in the string
(ii) mass per unit length of string
(iii) vibrating length of the string
Establish dimensionally the relation for frequency.
Defineangular velocity and angular displacement.
A bus starting from rest moves with a uniform acceleration of 0.2 ms-2 for 3 minutes. Find the speed and distance travelled.
Answers
6%

10 J
\(\frac { 1 }{ 2 } \frac { { { I }_{ b }{ I }_{ b } } }{ ({ I }_{ a }+{ I }_{ b }) } { \omega }^{ 2 }\)
\({g\over 2}\)
wire 1
adiabatic
T1 > T2
\(T=4\pi \sqrt { m\left( \frac { 1 }{ { k }_{ 1 } } +\frac { 1 }{ { k }_{ 2 } } \right) } \)
(B), (C) and (A)
3.26
106 \(\mu\)m
internal energy decreases
The principle of moments states that, "when an object is in equilibrium, the sum of the anti clockwise moments about a turning point must be equal to the sum of the clockwise moments.
1. Law of orbits
Each planet moves around the Sun in an elliptical orbit with the Sun at one of the foci.
2. Law of area
The radial vector (line joining the Sun to a planet) sweeps equal areas in equal intervals of time.
3. Law of period
The square of the time period of revolution of a planet around the Sun in its elliptical orbit is directly proportional to the cube of the semi major axis of the ellipse. It can be written as :
\(T^{2} \propto a^{3} \)
\(\frac{T^{2}}{a^{3}}=\text { constant. }\)
We know that \(\frac{F}{A}\) = Y\(\times \frac{\Delta L}{L}\)
\(\frac{\Delta{L}}{L}\)=\(\left( \frac { F }{ A } \right) \left( \frac { L }{ Y } \right) \)
\(=\left( \frac { 50 }{ 1.25\times { 10 }^{ -4 } } \right) \left( \frac { 10 }{ 4\times { 10 }^{ 10 } } \right) ={ 10 }^{ -4 }m\)
When we cut vegetables with a sharp knife the area of contact is less. Hence the stress applied becomes more \([Stress =\frac{\text { Force }}{\text { Area }}=\frac{F}{\Delta A}]\) the vegetables would be cut easily. So, it is so easy to cut vegetables with a sharp knife rather than a blunt knife.
Work done on the system (by the person while stirring), W = -30 kJ = -30,000J
Heat flowing out of the system,
Q = -5 kcal = 5\(\times\)4184 J =-20920 J
Using First law of thermodynamics
\(\Delta\)U = Q-W
\(\Delta\)U = -20,920 J - (-30,000) J
\(\Delta\)U = -20,920 J + 30,000 J = 9080 J
Here, the heat lost is less than the work done on the system, so the change in internal energy is positive.
PV diagram is a graph between pressure P and volume V of the system.
a. Periodic motion
b. Non-periodic motion
c. Periodic motion
(a) Bulk modulus
\(B =V\left|\frac{\Delta P}{\Delta V}\right|=\frac{100 \times 10^{3}}{0.005 \times 10^{-2}}=
\)
\(=\frac{100 \times 10^{3}}{5 \times 10^{-5}}=2000 \mathrm{MPa}, \text { where }\)
MPa is mega pascal
(b) Speed of sound in water is
\(v=\sqrt { \frac { B }{ \rho } } =\sqrt { \frac { { 200\times 10 }^{ 6 } }{ 1000 } } =1414 \ ms^{-1}\)
(i) Physics has a large scope as it covers a various physical quantities. (Length, mass, time, energy).
(ii) It deals with the Macroscopic group (Mechanics, electrodynamics, thermodynamics and optics) and microscopic group (Quantum physics).
Example:
Range of time scale (astronomical scale to microscopic group (Quantum physics).
Radius of the sphere R = 1.5 cm
mass m = 0.038 kg
Volume of the sphere V = \(\frac{4}{3}\pi{R^2}\)
= \(\frac{4}{3}\)\(\times\)(3.14)\(\times\)(1.5\(\times\)10-2)3 = 1.413\(\times\)10-5m3
Therefore, density
\(\rho =\frac { m }{ V } =\frac { 0.038kg }{ 1.413\times { 10 }^{ -5 }{ m }^{ 3 } } =2690{ kg \ m }^{ -3 }\)
Hence, the specific gravity of the sphere
\(=\frac { 2690 }{ 1000 } =2.69\)
The water in the tumbler is at a higher temperature than the bucket of normal water. But the bucket of normal water has larger internal energy than the hot water in the tumbler. This is because the internal energy is an extensive variable and it depends on the size or mass of the system.
Even though the bucket of normal water has larger internal energy than the tumbler of hot water, heat will flow from water in the tumbler to the water in the bucket. This is because heat flows from a body at higher temperature to the one at lower temperature and is independent of internal energy of the system.
Once the heat is transferred to an object it becomes internal energy of the object. The right way to say is ‘object has certain amount of internal energy’. Heat is one of the ways to increase the internal energy of a system.
Thermal expansion is the tendency of matter to change in shape, area, and volume due to a change in temperature.
From (9.26) \(\lambda =\frac { 1 }{ \sqrt { 2 } \pi { nd }^{ 2 } } \)
We have to find the number density n By using ideal gas law
\(n=\frac { N }{ V } =\frac { P }{ KT } =\frac { { 101.3\times 10 }^{ 3 } }{ 1.381\times { 10 }^{ -23 }\times 300 } \)
= 2.449\(\times\)1025 molecues/m3
\(\lambda =\frac { 1 }{ \sqrt { 2 } \times \pi \times 2.449\times { 10 }^{ 25 }\times \left( 1.2\times { 1 }0^{ -10 } \right) ^{ 2 } } \)
\(=\frac { 1 }{ 15.65\times 10^{ 5 } } \)
λ = 0.63\(\times\)10−6m
a. The displacement of the particle executing simple harmonic motion
y = A sin\(\omega t\)
Velocity of the particle is
v = A \(\omega\) cos \(\omega t\) = A \(\omega\) sin\(\left( \omega t+\frac { \pi }{ 2 } \right) \)
The phase difference between displacement and velocity is \(\frac{\pi}{2}\)
b. The velocity of the particle is
v = A \(\omega\) cos \(\omega t\)
Acceleration of the particle is
a = -A\({ \omega t }^{ 2 }\) sin \(\omega t\) = A \({ \omega }^{ 2 }\)cos \(\left( \omega t+\frac { \pi }{ 2 } \right) \)
The phase difference between velocity and acceleration is\(\frac{\pi}{2}\)
c. The displacement of the particle is
y = A sin\(\omega t\)
Acceleration of the particle is
a = − A \({ \omega }^{ 2 }\) sin ωt = A \({ \omega }^{ 2 }\) sin(\(\omega t\) + \(\pi\))
The phase difference between displacement and acceleration is \(\pi\) radian.
If an oscillator moves in a resistive medium its amplitude goes on decreasing then the motion of the oscillator is said to be damped oscillation.
Example: Electromagnetic oscillation in a tank circuit.
In an Octane, let the frequencies of be f, (f + n), (f + 2n), ......f + (N - 7)n
∴ Sum of two frequencies = 2f
f + (N - 1) n = 2f
(N - 1)n = 2f - f = f
∴ f = n(N - 1)
Fundamental quantities:
Fundamental quantities are quantities which cannot be expressed in terms of any other physical quantity.
Example: Quantities like length, mass, time, temperature are fundamental quantities.
Derived quantities:
Quantities that can be expressed in terms of fundamental quantity are called derived quantities.
Example: Quantities like area, volume, velocity are derived quantities.
1.496\(\times\)1011m is equivalent to 1 AU.
1 metere is equivalent to \({{1}\over{1.496\times{10}^{11}}}\)
= 0.6884\(\times\)10-11
= 6.68\(\times\)10-12 AU
In one more, 6.68\(\times\)10-12 astronomical units are present.
If two vectors which are inclined to each other are represented in magnitude and direction by the two adjacent sides of a triangle taken in order, then their resultant is the closing side of the triangle taken in the reverse orders.
Let us consider two vectors, \(\overrightarrow { A } \) and \(\overrightarrow { B } \) inclined an angle \(\theta\) with each other as shown in figure
To find the resultant, the head of the first vector \(\overrightarrow { A } \) is connected to the tail of the second vector \(\overrightarrow { B } \). Let \(\theta\) be the angle between them. The resultant vector \(\overrightarrow { R } \) is the line drawn connecting the tail of the first vector, \(\overrightarrow { A } \) to the head of the second vector \(\overrightarrow { B } \). Thus \(\overrightarrow { R } \) = \(\overrightarrow { A } \)+ \(\overrightarrow { B } \). The magnitude is \(\overrightarrow { R } \) (resultant) is given geometrically by the length of \(\overrightarrow { R } \)(\(\theta\)). The direction of the resultant is the angle between \(\overrightarrow { R } \) and \(\overrightarrow { A } \).
To find the magnitude of the resultant, the triangle ABN is obtained by extending OA to ON and drawing the perpendicular drop from B.
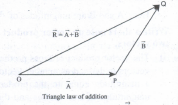
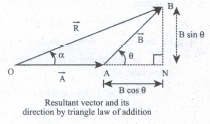
ABN is a right angled triangle. From the figure, \(\cos\ \theta={{AN}\over{B}}\ \therefore AN=B\ \cos\ \theta\)
\(\sin\ \theta={{BN}\over{B}}\ \therefore BN=B\ \sin\theta\)
For \(\triangle OBN,\) we have OB2 + ON2 + BN2
\(R^2=(A+B\ \cos\theta)^2+(B\sin\theta)^2\)
\(=A^2+B^2cos^2\theta+2AB\ \cos\theta+B^2\sin^2\theta\)
\(=A^2+B^2(\cos^2\theta+\sin^2\theta)+2AB\ \cos\theta\)
\( R\ =\sqrt{A^2+B^2+2AB \cos\theta}\)
Let \(\overrightarrow { R } \) makes an angle \(\alpha\) with \(\overrightarrow{A},\) then in \(\triangle OBN,\)
\(\tan\ \alpha={{BN}\over{ON}}+{{BN}\over{OA+AN}}=\frac{B \ sin \theta}{A +B\ cos \theta}\)
\(\therefore \alpha={\tan}^{-1}\left[ {{B\sin\theta}\over{A+B\cos\theta}} \right]\)
Work-Kinetic Energy Theorem
Work and energy are equivalents. This is true in the case of kinetic energy also. To prove this, let us consider a body of mass m at rest on a frictionless horizontal surface.
The work (W) done by the constant force (F) for a displacement (s) in the same direction is,
W = Fs
The constant force is given by the equation,
F = ma
The third equation of motion can be written as,
\(v^{2} =u^{2}+2 a s \)
\(a =\frac{v^{2}-u^{2}}{2 s}\)
Substituting for a in equation (2),
\(F=m\left(\frac{v^{2}-u^{2}}{2 s}\right)\)
Substituting equation (2), (1)
\(w=m\left(\frac{v^{2}}{2 s} s\right)-m\left(\frac{u^{2}}{2 s} s\right) \)
\(w=\frac{1}{2} m v^{2}-\frac{1}{2} m u^{2}\)
The expression for kinetic energy:
The term \(\left(\frac{1}{2} m v^{2}\right)\) in the above equation is the kinetic energy of the body of mass (m) moving with velocity(v).
\(K E=\frac{1}{2} m v^{2}\)
Kinetic energy of the body is always positive. From equations (4) and (5)
\(\Delta K E =\frac{1}{2} m v^{2}-\frac{1}{2} m u^{2} \)
\(\text {Thus, } W =\Delta K E\)
The expression on the right hand side (RHS) of equation (6) is the change in kinetic energy (\(\Delta\)KE) of the body.
This implies that the work done by the force on the body changes the kinetic energy of the body, This is called work-kinetic energy theorem.
The work-kinetic energy theorem implies the following.
1. If the work done by the force on the body is positive then its kinetic energy increases.
2. If the work done by the force on the body is negative then its kinetic energy decreases.
3. If there is no work done by the force on the body then there is no change in its kinetic energy, which means that the body has moved at constant speed provided its mass remains constant.
The angle 7.2 degree is equivalent to \(\frac{1}{8}\) radian. So \(\theta=\frac{1}{8} \mathrm{rad}\)
If S is the length of the arc between the cities of Syne and Alexandria and if R is radius of Earth, then
S = R\(\theta \)
= 500 miles
So radius of the Earth
\(\mathrm{R}=\frac{500}{\theta} \text { miles } \)
\(\mathrm{R}=500 \frac{\text { miles }}{1 / 8} \)
R = 4000 miles
1 mile is equal to 1.609 km. So, he measured the radius of the Earth to be equal to R = 6436 km, which is amazingly close to the correct value of 6378 km.
This is a thermodynamic process in which the volume of the system is kept constant. But pressure, temperature and internal energy continue to be variables.
The pressure-volume graph for an isochoric process is a vertical line parallel to pressure axis as shown in Figure.
The equation of state for an isochoric process is given by
\(P=\left( \cfrac { \mu R }{ V } \right) T\) ...(1)
Where \(\left( \cfrac { \mu R }{ V } \right) \)= constant
It is that the pressure is directly proportional to temperature. This implies that the P-T graph for an isochoric process is a straight line passing through origin.
If a gas goes from state (Pi,Ti) to (Pf, Tf) at constant volume, then the system satisfies the following equation
\(\cfrac { { P }_{ i } }{ { T }_{ i } } =\cfrac { { P }_{ f } }{ { T }_{ f } } \) ...(2)
For an isochoric processes, \(\triangle \)V = 0 and W = 0. Then the first law becomes
\(\triangle \)U = Q ....(3)
Implying that the heat supplied is used to increase only the internal energy. As a result the temperature increases and pressure also increases.
Suppose a system loses heat to the surroundings through conducting walls by keeping the volume constant, then its internal energy decreases. As a result the temperature decreases; the pressure also decreases.
1. When food is cooked by closing with a lid as shown in figure.
When food is being cooked in this closed position, after a certain time you can observe the lid is being pushed upwards by the water steam. This is because when the lid is closed, the volume is kept constant. As the heat continuously supplied, the pressure increases and water steam tries to push the lid upward's.
2. In automobiles the petrol engine undergoes four processes. First the piston is adiabatically compressed to some volume as shown in the Figure (a). In the second process (Figure (b)), the volume of the air-fuel mixture is kept constant and heat is being added. As a result the temperature and pressure are increased. This is an isochoric process. For a third stroke (Figure (c)) there will be an adiabatic expansion and fourth stroke again isochoric process by keeping the piston immoveable (Figure (d)).
Let us consider a massless spring with stiffness constant or force constant k attached to a ceiling as shown in figure. Let the length of the spring before loading mass m be L. If the block of mass m is attached to the other end of spring, then the spring elongates by a length l. Let F1 be the restoring force due to stretching of spring. Due to mass m, the gravitational force acts vertically downward. We can draw free body diagram for this system as shown in figure. When the system is under equilibrium,
\(\mathrm{F}_{1}+\mathrm{mg}=0\) .....(1)
But the spring elongates by small displacement l, therefore,
\(F_{1} \propto l \Rightarrow F_{1}=-\mathrm{k} l\) ......(2)
Substituting equation in equation, we get
\(-\mathrm{k} l+\mathrm{mg} =0
\)
\(\mathrm{mg} =\mathrm{k} l
\) or
\(\frac{m}{k} =\frac{l}{g} \) .....(3)
Suppose we apply a very small external force on the mass such that the mass further displaces downward by a displacement y, then it will oscillate up and down. Now, the restoring force due to this stretching of spring (total extension of spring is y+l) is
\(\mathrm{F}_{2} \propto(y+l)
\)
\(\mathrm{F}_{2}=-\mathrm{k}(\mathrm{y}+l)=-\mathrm{ky}-\mathrm{k} l\) ......(4)
Since, the mass moves up and down with acceleration \(\frac{d^{2} y}{d t^{2}},\) by drawing the free body diagram for this case, we get
\(-\mathrm{k} y-\mathrm{k} l+m g=\mathrm{m} \frac{d^{2} y}{d t^{2}}\) .....(5)
The net force acting on the mass due to this stretching is
\(\mathrm{F}=\mathrm{F}_{2}+m g
\)
\(\mathrm{~F}=-\mathrm{k} y-\mathrm{k} l+m g\) .....(6)
The gravitational force opposes the restoring force. Substituting equation in equation, we get
\(\mathrm{F}=-\mathrm{k} y-\mathrm{k} l+\mathrm{k} l=-\mathrm{k} y\) .....(7)
Applying Newton's law, we get
\(m \frac{d^{2} y}{d t^{2}} =-\mathrm{k} y
\)
\(\frac{d^{2} y}{d t^{2}} =-\frac{k}{m} y\) ......(8)
The above equation is in the form of simple harmonic differential equation. Therefore, we get the time period as
\(\mathrm{T}=2 \pi \sqrt{\frac{m}{k}} \text {second }\) ........(9)
The time period can be rewritten using equation
\(\mathrm{T}=2 \pi \sqrt{\frac{m}{k}}=2 \pi \sqrt{\frac{l}{g}} \text { second }\) ......(10)
The acceleration due to gravity g can be computed from the formula
\(g=4 \pi^{2}\left(\frac{l}{T^{2}}\right) m s^{-2}\) ......(11)
(a) Closed organ pipes:
Closed organ is a pipe with one end closed and the other end open. If one of a pipe is closed, the wave reflected at this closed end is 180o out of phase with the incoming wave. Thus there is no displacement of the
particles at the closed end. Therefore, nodes are formed at the closed end and anti-nodes are formed at open end.
Let us consider the simplest mode of vibration of the air column called the fundamental mode. Anti-node is formed at the open end and node at closed end. From the figure let L be the length of the tube and λ be the wavelength of the wave produced. For the fundamental mode of vibration, we have,
\(L={\lambda_1\over4}(or)\lambda_1=4L\)
The frequency of the note emitted is
\(f_1={v\over \lambda_1}={v\over 4L}\)
which is called the fundamental note.
The frequencies higher than fundamental frequency can be produced by blowing air strongly at open end. Such frequencies are called overtones.
The figure (2) shows the second mode of vibration having two nodes and two anti-nodes
4L = 3λ2
\(L={3⋋_2\over 2}or\ ⋋_2={4L\over 3}\)
The frequency for this
\(f_1={v\over \lambda_2}={3v\over 4L}=3f_1\)
is called first over tone, since here, the frequency is three times the fundamental frequency it is called third harmonic.
The Figure (3) shows third mode of vibration having three nodes and three anti-nodes.
We have 4L= 5⋋3
\(L={5\lambda_3\over4}\ or\ \lambda_3={4L\over 5}\)
The frequency
\(f_3={v\over \lambda _3}={5v\over 4L}=5f_1\)
is called second over tone, and since n = 5 here, this is called fifth harmonic. Hence, the closed organ pipe has only odd harmonics and frequency of the nth harmonic is f n = (2n + 1)f1. Therefore, the frequencies of harmonics are in the ratio
f1:f2:f3:f4:.... = 1:3:5:7:....
(b) Open organ pipe:
Consider the picture of a flute, shown in Figure. It is a pipe with both the ends open. At both open ends, anti-nodes are formed. Let us consider the simplest mode of vibration of the air column called fundamental mode. Since anti-nodes are formed at the open end, a node is formed at the mid-point of the pipe
From Figure (5), if L be the length of the tube, the wavelength of the wave produced is given by
\(L={\lambda_1\over 2}or\ {\lambda_1=2L}\)
The frequency of the note emitted is
\(f_1={v\over \lambda_1}={v\over 2L}\)
which is called the fundamental note.
The frequencies higher than fundamental frequency can be produced by blowing air strongly at one of the open ends. Such frequencies are called overtones.
The Figure (6) shows the second mode of vibration in open pipes. It has two mode of vibration in open pipes. It has two nodes and three anti-nodes, and therefore,
L= ⋋2 or ⋋2= L
The frequency
\(f_2={v\over \lambda_2}={v\over L}=2\times{v\over 2L}=2f_1\)
is called first over tone. Since n = 2 here, it is called the second harmonic.
The Figure (7) above shows the third mode of vibration having three nodes and four anti-nodes
\(L={3\over2}\lambda,\ or\ \lambda_3={2L\over 3}\)
The frequency
\(f_3={v\over \lambda_3}={3v\over 2L}=3f_1\)
is called second over tone. Since n = 3 here, it is called the third harmonic.
Hence, the open organ pipe has all the harmonics and frequency of nth harmonic is f n = nf1, Therefore, the frequencies of harmonics are in the ratio
f1 : f2 : f3 : f4 :... = 1 : 2 : 3 : 4 : ....
As F \(=G{m_1m_2\over r^2}\)
\(G={F.r^2\over m_1m_2}\)
\([G]={{MLT}^{-2}.L^2\over MM}={M}^{-1}L^3{T}^{-2}\)
\(\therefore\) a = -1, b ~ 3, c = -2
| CGS units | SI units |
|---|---|
| n1 = 6.67\(\times\) 10-8 | n2 =?, |
| m1=1g | m2 = 1 kg=1000 g |
| L1 = 1cm | L2 = 1 cm = 100cm |
| T1=1s | T2=1s |
\(\therefore\) \(n_2=n_1{\left[ {M_1 \over M_2} \right]}^{a}{\left[ {L_1 \over L_2} \right]}^{b}{\left[ {T_1 \over T_2} \right]}^{c}\)
\(=6.67\times{10}^{-8}{\left[ {{1\over 1000}} \right]}^{-1}{\left[ {{1\over 100}} \right]}^{3}\left[ {1\over 1} \right]^{-2}=6.67\times{10}^{}-11\)
Hence in SI units, G = 6.67\(\times\)10-11Nm2 kg-2
n\(\propto\) IaTbmc, [I] = [MoL1To]
[T] = [M1L1T-2] (force)
[M] = [M1L-1To]
[Mo LoT-1] = [MoL1To]a [M1L1T-2]b [MoL-1To]C
b + c = 0
a + b - c = 0
-2b = -1 \(\Rightarrow\) b = \(1\over2\)
c =\(-{1\over2}a=1\)
n\(\propto\) \({1\over l}{\sqrt{T\over m}}\)
| Rule | Example |
| If the digit to be dropped is smaller than 5, then the preceding digit should be left unchanged. | 7.32 is rounded off to 7.3 8.94 is rounded off to 8.9 |
| If the digit to be dropped is greater than 5, then the preceding digit should be increased by 1. | 17.26 is rounded off to 17.3 11.89 is rounded off to 11.9 |
| If the digit to be dropped is 5 followed by digits other than zero, then the preceding digit should be raised by 1. | 7.352, on being rounded off to first decimal becomes 7.4 18.159 on being rounded off to first decimal, become 18.2 |
| If the digit to be dropped is 5 or 5 followed by zeros, then the preceding digit is not changed if it is even. | 3.45 is rounded off to 3.4 8.250 is rounded off to 8.2 |
| If the digit to be dropped is 5 or 5 followed by zeros, then the preceding digit is raised by 1 if it is odd. | 3.35 is rounded off to 3.4 8.350 is rounded off to 8.4 |
Initial velocity 'u' =0
Acceleration 'a' = 0.2 ms-2
Time (t) = 3 minutes = 180 seconds.
Speed of a bus, v = u + at
= 0 + 0.2\(\times\) 180
= 36 m/s.
Displacement s = ut+\(\frac { 1 }{ 2 } { at }^{ 2 }\)
\(=0\times 180+\frac { 1 }{ 2 } \times 0.2\times ({ 180) }^{ 2 }\)
\(=0+\frac { 1 }{ 2 } \times 0.2\times 32400\)
= 3240 m
=3.24 km











