The temperature T in celsius in a long rod of length 10 m, insulated at both ends, is a function of length x given by T = x(10 − x). Prove that the rate of change of temperature at the midpoint of the rod is zero.

A person learnt 100 words for an English test. The number of words the person remembers in t days after learning is given by W(t) = 100 × (1− 0.1t)², 0 ≤ t ≤ 10. What is the rate at which the person forgets the words 2 days after learning?

A particle moves so that the distance moved is according to the law s(t) = \(s(t)=\frac{t^{3}}{3}-t^{2}+3\). At what time the velocity and acceleration are zero.

The price of a product is related to the number of units available (supply) by the equation Px + 3P −16x = 234, where P is the price of the product per unit in Rupees(Rs) and x is the number of units. Find the rate at which the price is changing with respect to time when 90 units are available and the supply is increasing at a rate of 15 units/week.

A particle moves along a straight line in such a way that after t seconds its distance from the origin is s = 2t² + 3t metres.

Find the equations of tangent and normal to the curve y = x² + 3x − 2 at the point (1, 2)

Find the slope of the tangent to the following curves at the respective given points
y = x⁴ + 2x² − x at x = 1

Find the slope of the tangent to the curves at the respective given points.
x = a cos³ t, y = b sin³ t at t = \(\frac { \pi }{ 2 } \)

Find the point on the curve y = x² − 5x + 4 at which the tangent is parallel to the line 3x + y = 7.

Find the tangent and normal to the following curves at the given points on the curve
y = x² − x⁴ at (1, 0)

Find the tangent and normal to the following curves at the given points on the curve
y = x⁴ + 2e^x at (0, 2)

Find the tangent and normal to the following curves at the given points on the curve
y = x sin x at \(\left( \frac { \pi }{ 2 } ,\frac { \pi }{ 2 } \right) \)

Find the tangent and normal to the following curves at the given points on the curve
x = cos t, y = 2sin t² at t = \(\frac { \pi }{ 3 } \)

Compute the value of 'c' satisfied by the Rolle’s theorem for the function f (x) = x² (1 - x)², x ∈ [0,1]

Find the values in the interval \((\frac{1}{2},2)\) satisfied by the Rolle's theorem for the function \(f(x)=x+\frac{1}{x}, x\in[\frac{1}{2},2]\)

Compute the value of 'c' satisfied by Rolle’s theorem for the function \(f(x)=log(\frac{x^{2}+6}{5x})\) in the interval [2, 3]

Without actually solving show that the equation x⁴+2x³-2 = 0 has only one real root in the interval (0, 1).

Prove that there is a zero of the polynomial \(2x^{3}-9x^{2}-11x+12\) in the interval (2, 7) given that 2 and 7 are the zeros of the polynomial \(x^{4}-6x^{3}-11x^{2}+24x+28\)

A truck travels on a toll road with a speed limit of 80 km/hr. The truck completes a 164 km journey in 2 hours. At the end of the toll road the trucker is issued with a speed violation ticket. Justify this using the Mean Value Theorem.

Suppose f(x) is a differentiable function for all x with f'(x) ≤ 29 and f(2) = 17. What is the maximum value of f(7)?

Prove, using mean value theorem, that \(|sin \alpha-sin\beta|\le |\alpha-\beta|, \alpha, \beta \in R\)

A thermometer was taken from a freezer and placed in a boiling water. It took 22 seconds for the thermometer to raise from −10°C to 100°C. Show that the rate of change of temperature at some time t is 5°C per second.

Explain why Rolle’s theorem is not applicable to the following functions in the respective intervals.
\(f(x)=|\frac{1}{x}|, x\in [-1,1]\)

Explain why Rolle’s theorem is not applicable to the following functions in the respective intervals.
\(f(x)=tan x,x \in [0, \pi]\)

Explain why Rolle’s theorem is not applicable to the following functions in the respective intervals.
\(f(x)=x-2logx, x\in [2,7]\)

Using the Rolle’s theorem, determine the values of x at which the tangent is parallel to the x -axis for the following functions:
f(x) = x² − x, x ∈ [0, 1]

Using the Rolle’s theorem, determine the values of x at which the tangent is parallel to the x -axis for the following functions:
\(f(x)=\frac{x^{2}-2x}{x+2}, x\in [-1,6]\)

Using the Rolle’s theorem, determine the values of x at which the tangent is parallel to the x -axis for the following functions:
\(f(x)=\sqrt{x}-\frac{x}{3}, x\in [0,9]\)

Explain why Lagrange’s mean value theorem is not applicable to the following functions in the respective intervals:
f(x) = \(\frac { x+1 }{ x } \), x ∈ [-1, 2]

Explain why Lagrange’s mean value theorem is not applicable to the following functions in the respective intervals
f(x) = |3x + 1|, x ∈ |-1, 3|

Find intervals of concavity and points of inflexion for the following functions
f(x) = x(x - 4)³

Find intervals of concavity and points of inflexion for the following function:
f(x) = sin x + cos x, 0 < x < 2

Find intervals of concavity and points of inflexion for the following function:
\(f(x)=\frac { 1 }{ 2 } \left( { e }^{ x }-{ e }^{ -x } \right) \)

Write the Maclaurin series expansion of the following function
e^x

Write the Maclaurin series expansion of the following function
sin x

Write the Maclaurin series expansion of the following function
cos x

Write the Maclaurin series expansion of the following function
log(1 - x); -1 ≤ x < 1

Write the Maclaurin series expansion of the following function
tan^-1(x); -1 ≤ x ≤ 1

Write the Maclaurin series expansion of the following function:
cos² x

Expand the polynomial f (x) = x² - 3x + 2 in powers of x - 1

Evaluate : \(\underset{x\rightarrow 1^{-}}{lim}(\frac{log(1-x)}{cot(\pi x)})\).

Evaluate: \(\underset{x\rightarrow 0^{+}}{lim}(\frac{1}{x}-\frac{1}{e^{x}-1})\).

Using the l’Hôpital Rule prove that, \(\underset{x\rightarrow 0^{+}}{lim}(1+x)^{\frac{1}{x}}=e\)

Evaluate: \( (\underset{x\rightarrow \infty}{lim}(1+2x)^{\frac{1}{2log\ x}}\)

Evaluate: \(\underset{x \rightarrow1}{lim} \ x^{\frac{1}{1-x}}\)

Evaluate the following limit, if necessary use l ’Hôpital Rule
\(\underset { x\rightarrow 0 }{ lim } \frac { 1-cosx }{ { x }^{ 2 } } \)

Evaluate the following limit, if necessary use  l ’Hôpital Rule
\(\underset { x\rightarrow \infty }{ lim } \frac { { 2x }^{ 2 }-3 }{ { x }^{ 2 }-5x+3 } \)

Evaluate the following limit, if necessary use l ’Hôpital Rule
\(\underset { x\rightarrow \infty }{ lim } \frac { x }{ logx } \) 

Evaluate the following limit, if necessary use  l ’Hôpital Rule
\(\underset { x\rightarrow \frac { { \pi }^{ - } }{ 2 } }{ lim } \frac { secx }{ tanx } \)

Evaluate the following limit, if necessary use l ’Hôpital Rule
\(\underset { x\rightarrow \infty }{ lim } { e }^{ -x }\sqrt { x } \)

Evaluate the following limit, if necessary use l ’Hôpital Rule
\(\underset { x\rightarrow 0 }{ lim } \left( \frac { 1 }{ sinx } -\frac { 1 }{ x } \right) \)

Evaluate the following limit, if necessary use l ’Hôpital Rule
\(\underset { x\rightarrow { 1 }^{ + } }{ lim } \left( \frac { 2 }{ { x }^{ 2 }-1 } -\frac { x }{ x-1 } \right) \)

Evaluate the following limit, if necessary use l ’Hôpital Rule
\(​​​​​​\underset { x\rightarrow \infty }{ lim } \ { \left( 1+\frac { 1 }{ x } \right) }^{ x }\)

Evaluate the following limit, if necessary use l ’Hôpital Rule 
\(\underset { x\rightarrow { \frac { \pi }{ 2 } } }{ lim } { \left( sinx \right) }^{ tanx }\)

Evaluate the following limit, if necessary use l’Hôpital Rule
\(\underset { x\rightarrow { { 0 }^{ + } } }{ lim } (cos{ x })^{ \frac { 1 }{ { x }^{ 2 } } }\)

Find the local extrema for the following function using second derivative test:
f(x) = -3x⁵ +5x³

Find the local extrema for the following function using second derivative test:
f(x) = x logx

If an initial amount A₀ of money is invested at an interest rate r compounded n times a year, the value of the investment after t years is \(A={ A }_{ 0 }{ \left( 1+\frac { r }{ n } \right) }^{ nt }\). If the interest is compounded continuously, (that is as n ➝∞), show that the amount after t years is A = A_oe^rt.

Find the local extrema for the following function using second derivative test:
f(x) = x² e^-2x

Find the absolute maximum and absolute minimum values of the function f (x) = 2x³ + 3x² −12x on [−3, 2]

Find the absolute extrema of the function f (x) = 3cos x on the closed interval \([0,2\pi]\)

Find the intervals of monotonicity and hence find the local extrema for the function f(x) = x² − 4x + 4

Prove that the function f(x) = x − sin x is increasing on the real line. Also discuss for the existence of local extrema.

Discuss the monotonicity and local extrema of the function \(f(x)=log(1+x)-\frac{x}{1+x},x>-1\) and hence find the domain where, \(log(1+x)>\frac{x}{1+x}\)

Determine the intervals of concavity of the curve y = 3+ sin x .

Find the intervals of monotonicity and local extrema of the function f(x) = x log x + 3x.

Find the intervals of monotonicity and local extrema of the function \(f(x)=\frac{1}{1+x^{2}}\)

Find the asymptotes of the curve \(f(x)=\frac { { 2x }^{ 2 }-8 }{ { x }^{ 2 }-16 } \)

We have a 12 square unit piece of thin material and want to make an open box by cutting small squares from the corners of our material and folding the sides up. The question is, which cut produces the box of maximum volume?

Find the local maximum and minimum of the function x² y² on the line x + y = 10

If f is continuous on a closed interval [a,b] , and c is any number between f (a) and f (b) inclusive, then there is at least one number x in the closed interval [a,b] , such that f (x) = c .

Let f (x) be continuous on a closed interval [a,b] and differentiable on the open interval (a,b) If f (a) = f (b) , then there is at least one point c∈(a,b) where f '(c) = 0.

Let f (x) be continuous in a closed interval [a,b] and differentiable in the open interval (a,b) (where f (a), f (b) are not necessarily equal). Then there exist at least one point c∈ (a,b) such that,
\(f^{\prime}(c)=\frac{f(b)-f(a)}{b-a}\)

If f (x) is continuous in closed interval [a,b] and differentiable in open interval (a,b) and if f'(x) > 0, x ∈ (a,b) , then for, x₁, x₂ ∈ [ a, b ], such that x₁ < x₂ we have, f (x₁) < f (x₂ ) 

(a) Taylor’s Series
Let f (x) be a function infinitely differentiable at x = a . Then f (x) can be expanded as a series, in an interval (x − a, x + a), of the form
\(f(x)=\sum_{n=0}^{\infty} \frac{f^{(n)}(a)}{n !}(x-a)^{n}=f(a)+\frac{f^{\prime}(a)}{1 !}(x-a)+\cdots+\frac{f^{(n)}(a)}{n !}(x-a)^{n}+\cdots\)
(b) Maclaurin’s series
If a = 0, the expansion takes the form
\(f(x)=\sum_{n=0}^{\infty} \frac{f^{(n)}(0)}{n !} x^{n}=f(0)+\frac{f^{\prime}(0)}{1 !} x+\cdots+\frac{f^{(n)}(0)}{n !} x^{n}+\cdots\)

Let \(\lim _{x \rightarrow \alpha} g(x)\) exist and let it be L and let f (x) be a continuous function at x = L . Then, \(\lim _{x \rightarrow \alpha} f(g(x))=f\left(\lim _{x \rightarrow \alpha} g(x)\right)\)

If the function f (x) is differentiable in an open interval (a,b) then we say,
(1) if
\(\frac{d}{d x}(f(x)) \geq 0, \forall x \in(a, b)\) then f (x) is increasing in the interval (a,b) ,
(2) if 
\(\frac{d}{d x}(f(x))>0, \forall x \in(a, b)\) then f (x) is strictly increasing in the interval (a,b) .
The proof of the above can be observed from Theorem 7.3.
(3) f (x) is decreasing in the interval (a,b) if
\(\frac{d}{d x}(f(x)) \leq 0, \forall x \in(a, b)\)
(4) f (x) is strictly decreasing in the interval (a,b) if
\(\frac{d}{d x}(f(x))<0, \forall x \in(a, b)\)

If f ( x) is continuous on a closed interval [a,b] , then f has both an absolute maximum and an absolute minimum on [a,b] .

If f (x) has a relative extremum at x = c then c is a critical number. Invariably there will be critical numbers of the function obtained as solutions of the equation f'(x) = 0 or as values of x at which f′(x) does not exist.

Let (c, f (c)) be a critical point of function f (x) that is continuous on an open interval I containing c . If f(x) is differentiable on the interval, except possibly at c , then f (c) can be classified as follows: (when moving across the interval I from left to right) 
(i) If f ′(x) changes from negative to positive at c, then f (x) has a local minimum f (c) .
(ii) If f ′(x) changes from positive to negative at c, then f (x) has a local maximum f (c) .
(iii) If f ′(x) is positive on both sides of c or negative on both sides of c , then f (c) is neither a local minimum nor a local maximum.

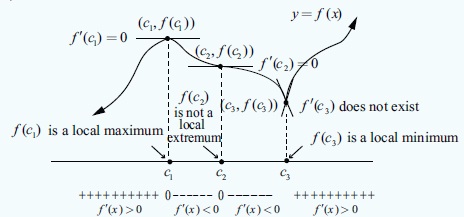
(i) If f ''(x) > 0 on an open interval I , then f (x) is concave up on I .
(ii) If f ''(x) < 0 on an open interval I , then f (x) is concave down on I .

(i) If f ′′(c) exists and f ′′(c) changes sign when passing through x = c, then the point (c, f (c)) is a point of inflection of the graph of f .
(ii) If f ′′(c) exists at the point of inflection, then f ''(c) = 0 .

Suppose that c is a critical point at which f '(c) = 0, that f ′(x) exists in a neighborhood of c , and that f ''(c) exists. Then f has a relative maximum value at c if f ''(c) < 0 and a relative minimum value at c if f ''(c) > 0 . If f ''(c) = 0 , the test is not informative.